Folks
I am confused about datum shift. In the counterbore stack, we see them bilateral in Line D & P. But in the other stack, they are bilateral unequal, Line E. Is 0.1/.005 come from the perpendicularity control. Also, Where Line F, G, Line J, K come from. The position tol has been accounted already in Line B&N
thanks
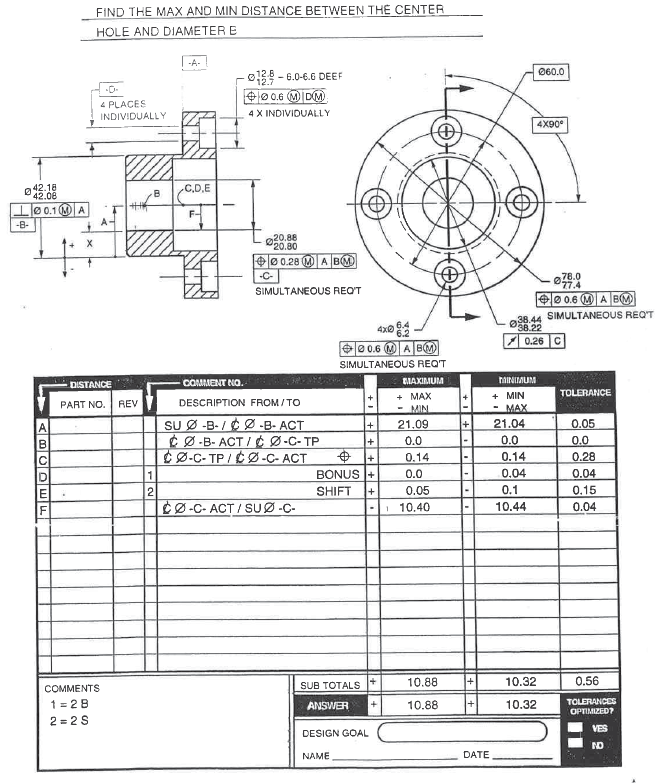
I am confused about datum shift. In the counterbore stack, we see them bilateral in Line D & P. But in the other stack, they are bilateral unequal, Line E. Is 0.1/.005 come from the perpendicularity control. Also, Where Line F, G, Line J, K come from. The position tol has been accounted already in Line B&N
thanks

