tangent to a curve

Hi,

How about posting your x,y data and the x,y point for the tangent.

Skip,

_{Just traded in my OLD subtlety...
for a NUance!}

maybe something like:



Dik

Improved version, both done in SMath Studio... a great program that's free:



Dik

I'm unclear how the sheet works for an arbitrary curve.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert! faq731-376 forum1529 Entire Forum list

IRS

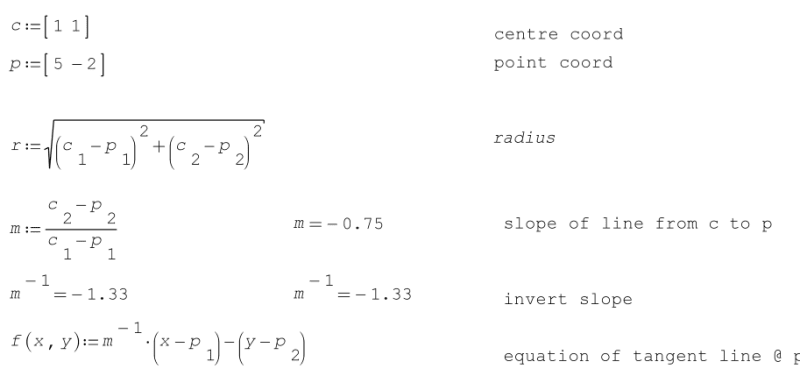
Works for a circle, or pretends it's a circle at the point of tangency (as any other tangent would). The two points are as follows, one defines the centre of the circle and the other defines the point of tangency. A line through them determines the radius of the circle and also the slope of the line. The inverse gives you a slope that matches the tangent.

The equation of the line is created by using a known point (ie, the tangent) and the slope of the tangent. From way back, y = mx + b, for a slope intercept definition of a line. By using the point data for the point of tangency, the function yields a 0 for the solution.

If it's just an arbitrary curve (not a circle) you have to determine the 'centre' for this and this would be the inverse to the derivative at the point. The centre of this 'circle' would fall anywhere on that line, but the point of tangency would remain.

Dik

I've attached the *.sm sheet, for anyone's info.

Dik

If you know the centre point for each point on any curve it's pretty easy to find the slope of the radius, and hence the slope of the tangent, but I assume the question relates to data where we just have the x,y values at points along some curve, and no other information.

There are of course infinitely many different curves that may be drawn through any finite number of points, but a reasonable approach is often to generate a cubic spline, which is a series of cubic curves where the slope and the curvature are continuous at each point.

See for an Excel spreadsheet that will generate a cubic spline through any xy data (listed with increasing x), and will return the y, slope and curvature for any x value.

Also search the blog for "cspline" for further articles with different types of cubic spline.

The latest version of the spreadsheet may be downloaded from:



Doug Jenkins
Interactive Design Services

Minor typo in the article: "Further details of the theory of cubicl splines"

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert! faq731-376 forum1529 Entire Forum list

Thanks IRStuff, and well spotted. Now fixed.

Doug Jenkins
Interactive Design Services

IDS:

For a circle, you only need to know the centre point of the circle and the tangent point... no other points are necessary.

Dik

Here's one I wrote nearly 30 years back... If someone has a basic compiler... have't had one for 20 years or more. Actually helped an aeronautical eng with airfoil curves using it.

Dik

For a circle, you only need to know the centre point of the circle and the tangent point... no other points are necessary.

Click to expand...

I know, but there is nothing in the OP to suggest that they are dealing with a circle.

Doug Jenkins
Interactive Design Services

I din't even think to a circle, but I was asking for any curve. Thanks for all your posts. I will read carefully in few days