Conservation of mass: control-volume approach

[George26]

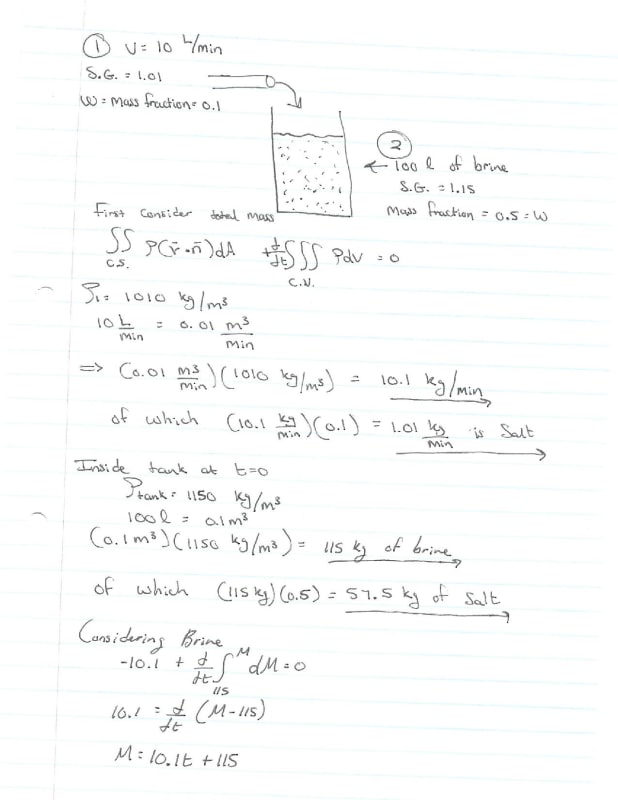
Hello, I'm currently struggling with the control volume problem in the attached picture. Can anybody give me guidence. I do get a first order differential equation and I usually just plug them into an online calculator but this one returns 'no solution'. I'm pretty sure I'm doing something incorrectly and I think it has to do with how I take into account the salt already in the tank. Thanks for the help in advance.

 

[onatirec]

I think you are making it way more complicated than necessary by introducing calculus and differential equations.

You're looking for total mass fraction of salt, which is just mass of salt over mass of brine, right?.

You seem to already have the total mass of salt = (1.01*60 + 57.5)kg, and the total mass of brine = (10.1*60 + 115)kg. Dividing one by the other should give you your answer...


Edit: your title mentions control volume, but the problem doesn't state that the tank is full or what the outflow is, etc. it looks to me like the simple mixing of two solutions...