The "purpose" for definition of FOS and AME (ASME Y14.5 2009) Conical /Tapered features 3

[dtmbiz]

Unfortunately the ”purpose” for the need to define certain and specific terms and concepts", which would be helpful in determining the “intent” of the standard’s definition regarding inclusion and /or exclusion for features considered in respect to those definitions and concepts. More specifically regarding FOS and AME for this discussion relative to conical and tapered features.

Here are my general points for discussion. I hope others will post theirs.

Purpose of FOS: (Feature of Size) My understanding of its purpose is to identify features that have center planes, axis and center points in order to locate and orient tolerance zones.

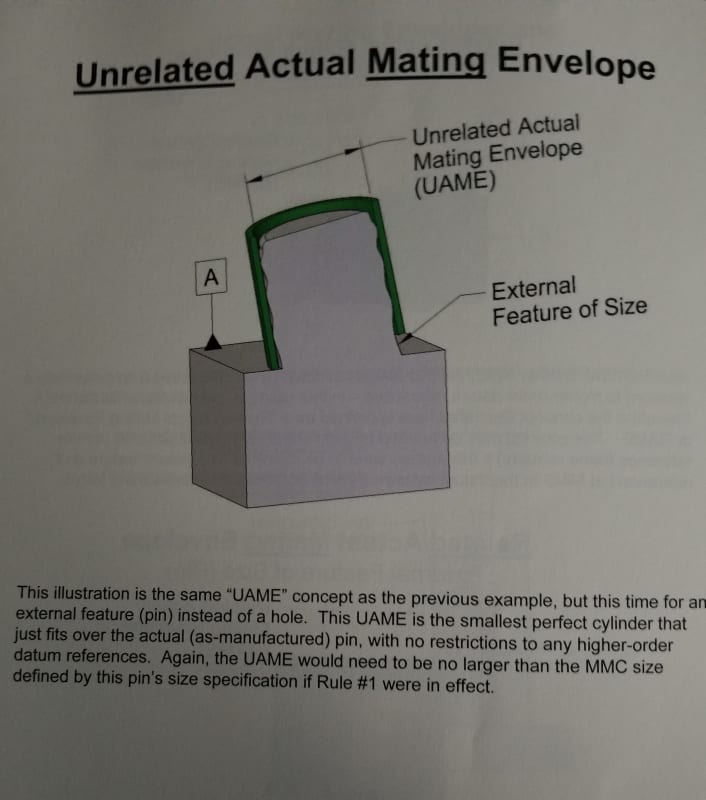
Purpose of AME: (Actual Mating Envelope) My understanding of its purpose is to establish a produced FOS’s actual location and orientation of it’s center plane, axis or center point by use of a AME Datum Simulator (gage) in order to verify compliance with the defined tolerance zone by comparing the true feature's vs. the produced feature's location and orientation of axis, center plane or center point.

It is also my understanding that applied geometric controls to an FOS, “other than size” can only be verified after the produced feature’s has been verified to be within size limits.

Conical and tapered feature’s: In a previous thread (thread1103-460248) there are arguments that conclude that these type features cannot be classified as features of size because in the case of a cone (conical feature) there is a limit to expansion or contraction about the apex, and similarly a limit to intersection of tapered surfaces beyond their intersection.


Disagreement with argument that 'conical and tapered surfaces are not FOSs because an AME cannot be defined":
Conical and tapered surfaces can and do have an AME in the physical world (vs a purist mathematical theoretical world) which can identify a produced FOS axis or center plane.
The AME’s Datum Simulator would not expand or contract beyond the limit of the apex of a conical surface or intersection of tapered surfaces whether or not they actually occurred within the size limits of the feature’s extent. Concluding that the apex of a conical surface and the intersection of tapered surfaces would be to one side of the tolerance limits and would be the minimum or maximum allowance which therefore would be the minimum or maximum limits of contraction or expansion of an AME. (internal / external feature). Expansion or contraction is limited to be within limits of size vs. infinite or unlimited

**** Also would like to mention in relevance to the FOS definitions, specifically around the standard's use of “may”, and the definitions of words ; may, must , will, shall in the engineering environment (English that is). “May” is a permission word that “allows” and is not mandatory. “Shall” is a “mandatory requirement” which is not used in definition of FOS

 

[Burunduk]

One additional thing that was perhaps misunderstood:

The expansion/contraction of a similar perfect (gage tolerances) counterpart contacts the produced feature's "high points" to define
a "mating size" per se (Actual Mating Envelope) for a produced feature. Not multiple variations of size through out the feature's surface imperfections.

When I said the simulator should cover a diametrical range I meant the theoretical span of local diameters of a cone. Nothing about imperfections.

 

[dtmbiz]

Burunduk

An AME is not an inspection for size, form, location, or orientation. It is an “envelope” that relates to
to how one specific uniquely produced feature would “mate” with another specific uniquely produced feature. It is not an instrument to specify or inspect features.

An AME is an envelope defined by results of a specific uniquely produced feature. “The envelope” includes the results of the uniquely produced feature’s size and form for an Unrelated AME. “The envelope” includes the results of the uniquely produced feature’s size, form, location, and orientation for a Related AME.

“High points” is a term used to reference the “extreme physical limits relative to how other physical elements of a feature would or would not contact the perfectly similar counterpart”. Variations in the context of an AME which is defined by a physical feature(s) i.e. “surface(s)” does not include the specified size within its specified tolerance. Regardless of the produced feature’s size, it is where
a similar “perfect” counterpart would make physical contact with the feature. This results in the fact that every AME is unique. No two are “perfectly alike”. It is based on a specific produced feature.

An analogy to ASME Y14.5 (any version) is to act as “referee” for application of ASME Y14.5
relative to approved terms, definitions, concepts and principles within the scope of the document.

“Theoretical” is used on a very limited basis. There is no “...theoretical span of local diameters…” referred to in Y14.5. Expand and contract are used in reference to physical features, within the limits of the feature’s specified tolerances.

 

[dtmbiz]

Burunduk

I clicked submit instead of preview for the previous post, so I did not get to add that I will provide a graphic of AME soon I hope.

 

[dtmbiz]

Burunduk,
Updates for:

An AME is not an inspection for size, form, location, or orientation. It is an “envelope” that relates to
to how one specific uniquely produced feature would “mate” with another specific uniquely produced feature. It is not an instrument to specify or inspect features.

Better wording:

An AME is not an inspection for size, form, location, or orientation. It is an “envelope” that relates to
to how one specific uniquely produced feature would “mate” when compared with another specific uniquely produced feature. It is not an instrument to determine specifications or inspect features.

“Theoretical” is used on a very limited basis. There is no “...theoretical span of local diameters…” referred to in Y14.5. Expand and contract are used in reference to physical features, within the limits of the feature’s specified tolerances.

Better wording:
“Theoretical” is used on a very limited basis within Y14.5. There is no “...theoretical span of local diameters…” referred to in Y14.5. Expand and contract are used in reference to physical features, within the limits of the feature’s specifications.

 

[Burunduk]

“Theoretical” is used on a very limited basis within Y14.5.

This has to be addressed first, as a basis for dealing with the other points: "Theoretical" simulators and envelopes are what the standard mostly defines and refers to. There is very little discussion of physical datum feature simulators or physical AME simulating devices in Y14.5.

“The envelope” includes the results of the uniquely produced feature’s size and form for an Unrelated AME.

Size - yes, form - not. The AME envelope of a cylindrical feature is always a perfect cylinder. The AME envelope of a width feature is two parallel theoretical planes that don't "include" any form error of the actual feature.

“The envelope” includes the results of the uniquely produced feature’s size, form, location, and orientation for a Related AME.

The Related AME is related to the applicable datum reference frame, not to the actual produced feature. Therefore it definitely doesn't "include the results" of the feature's form, location, and orientation.

This results in the fact that every AME is unique. No two are “perfectly alike”. It is based on a specific produced feature.

The geometry of the AME of a conical feature* is dependent only on the theoretical geometry specified for the feature in the drawing or CAD model. Therefore, the AME envelopes of 2 different produced physical features can certainly be identical.

* If it exists (opinions differ)

 

[Burunduk]

dtmbiz,
After re-reading your last post:
If by "including the results" of the form of the feature by an Unrelated AME you mean that only the envelope's size may change as a result of the actual features form error, then you are correct. If you mean that the AME "copies" the form error of the feature, I stand by my initial response.

Similarly, if you mean that only the related actual mating envelope's size "includes" the orientation, location, and form error of the feature, you are correct there too. If you mean that the envelope's orientation, location, and form are affected - I stand by my initial response.

About the conical features - I stand by my assertion that two different produced features can be simulated by an identical AME envelope (which of course doesn't have any form errors).

 

[dtmbiz]

Burunduk

It is "critical" to use terms and their definitions IAW Y14.5, anything else creates chaos in discussions.

My previous post regarding AME's is correct (however I did leave out orientation for UAME).

Your reply is incorrect on all points, which appears to be mainly due to the incorrect use of the term "Theoretical" and "it's place" within the context of Y14.5.

ALL GDT is applied to drawings and CAD models in order to produce physical parts.

Drawings and Cad models are not "theoretical".

Here is Merriam-Webster definition of "theoretical":

1: existing only in theory : HYPOTHETICAL
gave as an example a theoretical situation
2a: relating to or having the character of theory : ABSTRACT
b: confined to theory or speculation often in contrast to practical applications : SPECULATIVE
theoretical physics

Drawings and Cad models are representations of a "perfect part" containing a collection of features with specifications and relationships that define the desired manufactured physical part.
There is a big difference between a "theoretical part" and a "perfect part"
These representations are not referred to as "theoretical" IAW Y14.5. For indeed by definitions of Y14.5, they are referenced as; "true geometric form", "true profiles", Features, etc.

AME's are defined by "physical measurables" not "theoretical immeasurables".

 

[Burunduk]

Not getting into literal dissection of "theoretical", but my use of this term is the same as in the standard:

datum
feature simulator (theoretical): the theoretically perfect boundary used to establish a datum from a specified
datum feature.
NOTE: Whenever the term “datum feature simulator” is used in
this Standard, it refers to the theoretical, unless specifically otherwise indicated.

The principles in this Standard are based on theoretical datum feature simulators and do not take into account any tolerances or error in the physical datum feature simulators

 

[Belanger]

Mark Foster is AGI President and was the "1st ever" (GDTP S-0114) to pass the ASME administered professional exam.

Mark was not the "first ever." If I recall, there were about 60 to 80 of us in that room in Southgate MI who all took the test at the same time back in November of 1997.

John-Paul Belanger
Certified Sr. GD&T Professional (GDTP S-0109)

 

[dtmbiz]

Belanger

My mention of "names" was intended to give credit were credit is due relative to the posted graphics, which graphics I did not create.

The words 'you have issue with' came from the company's literature's exact wording.

The substance of the graphics is not diminished in any way regardless whether or not the referenced person is the "1st" or "one of the 1st" to receive ASME certification or even if they have no certification at all.

I have learned a valuable lesson though and that would be not to ever mention another's personal name or company. Potentially, those persons would not have opportunity to defend themselves in this forum.

My intent was to present graphics in order to help others more clearly understand my personal interpretations of Y14.5 wording and intent relative to the OP.

 

[Belanger]

Dtmbiz -- yes, I noticed the quotation marks in your post. Riffing on what 3DDave said, different experts can have different views on these gray areas, and it doesn't really matter how long they've been certified. The burden is on the standard to clarify things, otherwise we can only go by what is often called the extension of principles.

John-Paul Belanger
Certified Sr. GD&T Professional
Geometric Learning Systems

 

[dtmbiz]

Belanger,


To be clear the graphics that I posted were used to visualize "my understands and interpretations" regardless of the credentials of the graphic's creator.

Certainly understood that different people have different interpretations regarding Y14.5, however can those interpretations have clear connections to the written text and "intent" of Y14.5.

The emphasis on this thread was hopefully being restricted to an individual's interpretation based on the wording and language of ASME Y14.5 specifically
relative to the definitions FOS and AME. Having insight into "intent" can be useful in understanding "purpose".(see OP). I have not seen any official (ASME Y14.5) reasoning for "the purpose" of defining
some terms and definitions while not for others.

The closer one can get to the "intent" and purpose regarding aspects of Y14.5, hopefully then an individual's interpretations can be inline with the written standard's text.
Without understanding Y14.5 text's intent and purpose there cannot be any meaningful "extension of principle".

Threads can go so far off course at times, it would be of interest to me and possibly others how closely aligned are their conclusions based on specific Y14.5 text.
Then there are others (not referring to you personally) who apparently like to ignore the text and develop fiction based theories and interpretations.

 

[3DDave]

FOS is strictly defined in ASME Y14.5.1-1994 in such a way as to exclude tapered features entirely.

The pursuit of conical features as "features of size" is a long-standing tradition for "read between the lines" enthusiasts.

One would think that after (2019 - ~1945 = ~74 years) that if it was going to be recognized in the standard as a feature of size that geometry as fundamental as a straight or conical taper would have made it by now; instead the mathematical explanation standard excludes them entirely.

Essentially, the Math standard limits features of size to those which are bounded by two surfaces that are defined by the motion of constant diameter spheres (the least and greatest dimensions as directly toleranced), each moving along a spine such that the surface in question lies entirely between them. For external surfaces, the least sized spine does not need to be perfectly straight, but the least surface must lie within the surface developed by the greatest sphere.

 

[Burunduk]

The swept spheres definition defines size, it doesn't define the concept of a feature of size.
A feature that doesn't have one single size value that describes its entire surface (or collection of surfaces) may still be considered an irregular feature of size per Y14.5-2009 and have an actual mating envelope as defined in both Y14.5.1M-1994 and Y14.5-2009 standards.

 

[3DDave]

An irregular feature of size still accepts the sphere and spine. I don't care about the "AME"; I'm excluding cones/tapers as FOS by definition and therefore AME had no bearing. If a feature does not have a size it cannot be a feature of size. QED.

 

[Burunduk]

3DDave, how would you apply the spheres and spine concept in relation to the irregular feature of size from fig. 8-24 of Y14.5-2009?

 

[3DDave]

Per the 1994 standard, the 2009 example is not a feature of size. Not surprising that the committee values creating new training classes over rigorous math, but here we are.

The latest math standard just does hand waving, but still uses as the sole example a constant cross section, though it seems to fail to allow for an irregular feature the same latitude to excursion from MMB to allow the feature to depart location as much as the size is altered because (get this,2 it is funny) the irregular feature of size has no associated size.

Still - no support for features that are not constant cross section, especially cones.

 

[Burunduk]

Per the 1994 standard, the 2009 example is not a feature of size...

The latest math standard just does hand waving...

There you go.
If the swept spheres concept is what defines a feature of size for you, no irregular feature of size type (b) can be considered a feature of size, tapered or not.

 

[3DDave]

Someone in the committee wanted to apply position tolerancing to random shapes and -poof- everything that they wanted to apply it to was by fiat declared a feature of size in order to be compatible with the position tolerance definition.

Not just for me. For anyone who believes the math standard. If the latest math standard provided an explanation for tapers that would be different. Right now only constant profile items are IFOS.

I would have preferred the committee members to do the work to develop a better definition for position tolerance rather than crippling the definition of feature of size, but here we are.

For example, ISO allows a flat surface to have a position tolerance, but the ASME members only want a certain level of compatibility so they don't lose out and get replaced by the ISO. (Why have one training course when you can have three? One ISO, one ASME, and one for differences training?)

 

[Burunduk]

If I want to find out what can and what cannot be a feature of size, I turn to the feature of size definition in the relevant standard, not to the mathematical definition of size as a characteristic. The swept spheres concept's scope is to mathematically define how the size of a feature is determined, conformance to a specification of a directly toleranced dimension, and actual value. It does all that only for regular features of size because at the time it was formed there were no other types of features of size. I don't have access to the newer mathematical definition but since you say that not much has changed, Y14.5 is all we have for irregular features of size.