ISO axial circular runout and perpendicularity 1

[ANGIDDT]

An ISO drawing referencing ISO14405 E, contains an axial circular runout as shown in the picture (surface nominal perpendicular to the datum axis). As an addition a perpendicularity within a smaller tolerance value has been added on the same surface.
My question is: does it make sense? Or the newly added perpendicularity overrides the runout?
I know the Independency principle is enforced in ISO, but still, how the above combo could be valid?

What about ASME? Still think it's the same story, meaning a non-sensical combination (considering shown tolerance values)

 

[chez311]

In ASME perpendicularity and total runout of a flat face are equivalent. Circular runout is a slightly different animal, however circular runout larger than perpendicularity would be redundant as perpendicularity would control all circular elements to within its tighter tolerance.

A circular runout tighter than perpendicularity on the other hand would not be redundant. It would control each individual circular element within the tighter circular runout and the entire surface within the looser perpendicularity tolerance. Ie: the surface could be conical taking up the entire looser perpendicularity tolerance and still satisfy the tighter circular runout.

 

[pmarc]

There is no difference between ISO and ASME in this case, so what chez311 wrote is true in ISO too.

 

[dgallup]

I agree, the runout is redundant.

----------------------------------------

The Help for this program was created in Windows Help format, which depends on a feature that isn't included in this version of Windows.

 

[Tmoose]

https://cdn.shopify.com/s/files/1/0043/5804/5763/products/20181124_222444_1024x1024.jpg?v=1543118538

Strict perpendicularity.
"Axial" and "circular" runout immense.

 

[chez311]

Tmoose,

What exactly are you trying to show there? I assume this is the shift drum along which the shift forks in a transmission ride or something similar and that you are referring to the grooves in which the shift forks ride.

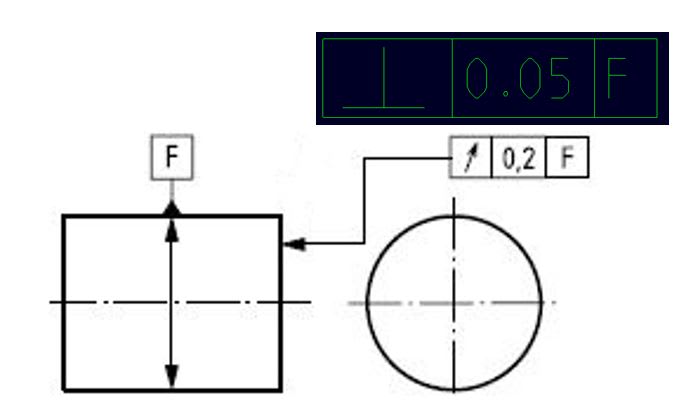
I'm fairly confident in my previous statement, and pmarc's confirmation solidifies that for me. The statement applied to a nominally flat surface which mirror's the OP's question. The surface you have introduced is not nominally flat, and I don't think surface perpendicularity/orientation makes sense on a feature which is not flat (no provisions per Y14.5 or Y14.5.1 for such an specification), perpendicularity applied to a cylindrical/width-shaped feature (or perhaps more generally - a feature which has a UAME aka FOS from which an axis/centerplane can be derived) would imply axis/centerplane perpendicularity at RFS (or surface if MMC is applied to the same type of feature) - your only option for the feature you have shown (approximately helical) would be perpendicularity of EACH ELEMENT.

If we assume you mean to compare the perpendicularity of EACH ELEMENT on the surface of the grooves to circular runout, I don't think you could apply circular runout to these grooves. Circular runout (and really total runout - this can be debated as theres no example of it, but the Y14.5.1 math standard definition is broad enough) can indeed be applied to profiles other than the nominally cylindrical and flat (ie: conical) but at least for circular runout the definition is pretty clear that such a feature must be able to be derived circular elements from the nominal geometry (cylindrical symmetry aka n-fold rotational symmetry where n=infinity). For total runout I'm less clear on whether the part must have cylindrical symmetry - certainly for "surfaces constructed at a right angle to the datum axis" only covers features which are nominally flat as per Y14.5.1-1994 section 6.7.2 "all points of the surface must lie in a zone bounded by two parallel planes perpendicular to the datum axis and separated by the specified tolerance" which would suggest only nominal planar features. To me the shape you presented would fall under "constructed at a right angle to the datum axis" and would thus be excluded as it is not nominally flat. If one were to try and cover it under 6.7.3 "Surfaces Constructed Around a Datum Axis" I'm not as certain - my gut says no but I'm interested in alternate views.

I think you're comparing apples and oranges anyway - even with a nominally flat surface perpendicularity of EACH ELEMENT would not be equivalent to perpendicularity of the entire surface, and definitely not to circular/total runout of said surface.