Incorrect formula for composite design in AISC?

[Perception]

Hello everyone,

I have a question about the formula to compute the moment capacity of a composite beam when the PNA is in the flange of the steel beam. I am perplexed by the formula given by AISC to compute moment capacity. First the stress distribution that AISC bases their formula off of is shown below.

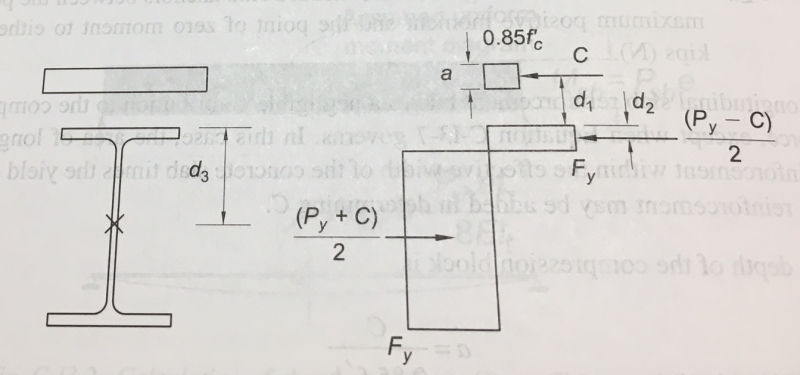
Based on the distribution above, AISC presents the formula below for the moment capacity of a composite section.

AISC is saying since both the portion of the flange in compression and the portion of the flange in tension have the term C/2 with opposite signs (see distribution above), the two terms cancel out. While this is true for the resultant force, the same logic can not be applied for summing moments. Since the two C/2 terms have different moment arms about the plastic neutral axis, the moment caused by the two terms will be different. From what I can tell steel textbooks seem to agree with this logic (at least McCormac does). I think this equation needs to be amended, or I need to refresh my statics... Am I missing something here? Is it possible that AISC decided the difference between moment arms is negligible?

I came across this discrepancy looking at the AISC Design Examples for composite section. Specifically, I was looking at example I.1 which looks at the design of a composite girder. In the example the plastic neutral axis is in the web, but the formula shown above is utilized to find the moment capacity.

A follow up question relating to the composite section moment capacities in the steel textbooks. Most of the textbooks double the compression force in the flange of the beam so they don't have to subtract anything from the tension force (i.e. T = FyAs instead of (FyAs - flange compression force) since I added more compression in the flange). I have considered both adding and not adding the additional compression in the flange, and both approaches give a different moment capacity due to the reasoning above (different moment arms). How do you choose to compute the moment capacity of a composite section when the plastic neutral axis is in the flange of the beam?

Thanks

 

[BAretired]

At a quick glance, I agree with you. Equation 13-10 appears to be overestimating the contribution of the steel beam.

BA

 

[nutte]

I've calculated the moment long-hand before, and I get the same answer as the equation. I haven't gone to the trouble of deriving the equation. Give it a try yourself and see.

 

[KootK]

While this is true for the resultant force, the same logic can not be applied for summing moments.

I think that the formula checks out. See the derivation below for the moment effect of the tensile forces in the steel about the d2 axis. The trick is to recognize that both C/2 forces are really halves of the same force C and, therefore, are located at the same vertical position. If that doesn't clear things up for you, report back and we'll kick it around some more.

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

 

[WillisV]

As long as the PNA is correctly located so that the sum of horizontal forces above is equal to the sum below (the definition of the PNA), you will get the same correct moment capacity no matter what location you choose as the datum to sum these forces about.