rfabdo89
Mechanical
- Sep 18, 2018
- 3
Here is the question:
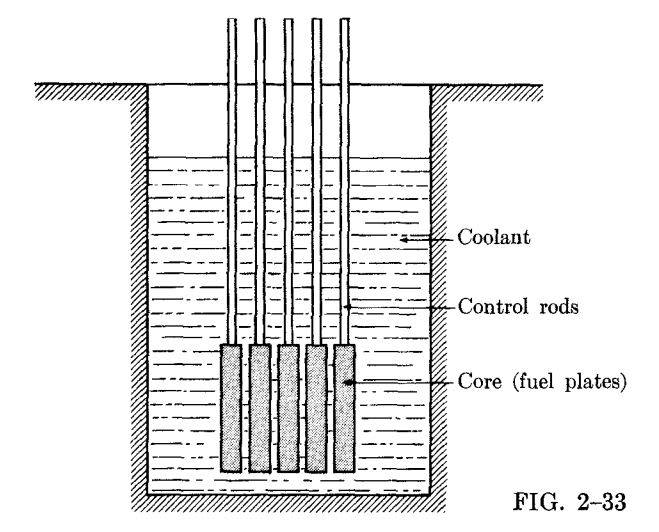
Consider a pool reactor (Fig. 2-33) whose core is constructed from a number of vertical fuel plates of thickness 2L. Initially the system has the uniform temperature T; then assume that the constant nuclear internal energy u"' is uniformly generated in these plates. The heat transfer coefficient between the plates and the coolant is h. The temperature of the coolant remains constant, and the thickness of the plates is small compared with other dimensions. Thus, if the end effects are neglected, the heat transfer may be taken to be one-dimensional. We wish to formulate the unsteady temperature problem of the reactor.
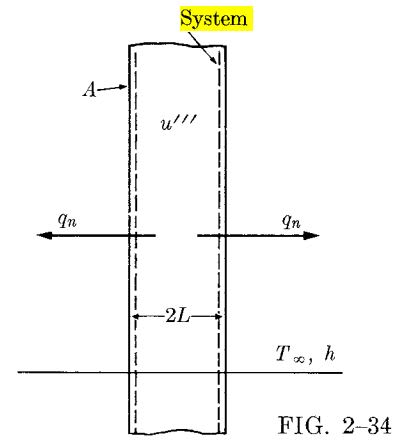
The author starts with the lumped formulation using this control volume:
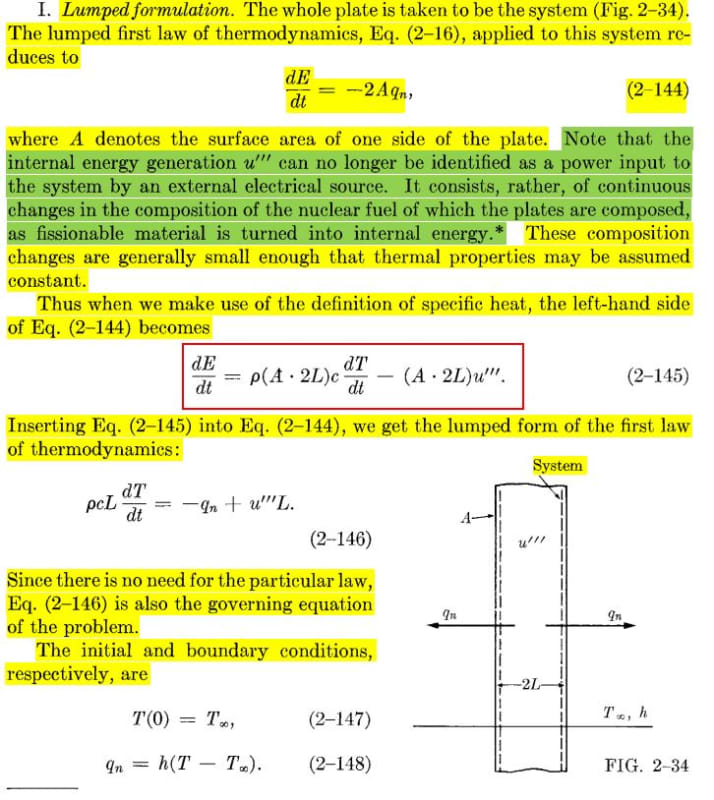
The lumped first law of thermodynamics applied to this system reduces to:
dE/dt=−2Aqn
Then the author states:
dE/dt=ρ(A.2L)cdT/dt−(A.2L)u′′′
u''' is the internal energy generation per unit volume.
Inserting the last equation into the first we get the lumped form:
ρcLdE/dt=−qn+u′′′L
My question is: Where did this equation come from:
dE/dt=ρ(A.2L)cdT/dt−(A.2L)u′′′
Here is an image:
Consider a pool reactor (Fig. 2-33) whose core is constructed from a number of vertical fuel plates of thickness 2L. Initially the system has the uniform temperature T; then assume that the constant nuclear internal energy u"' is uniformly generated in these plates. The heat transfer coefficient between the plates and the coolant is h. The temperature of the coolant remains constant, and the thickness of the plates is small compared with other dimensions. Thus, if the end effects are neglected, the heat transfer may be taken to be one-dimensional. We wish to formulate the unsteady temperature problem of the reactor.

The author starts with the lumped formulation using this control volume:

The lumped first law of thermodynamics applied to this system reduces to:
dE/dt=−2Aqn
Then the author states:
dE/dt=ρ(A.2L)cdT/dt−(A.2L)u′′′
u''' is the internal energy generation per unit volume.
Inserting the last equation into the first we get the lumped form:
ρcLdE/dt=−qn+u′′′L
My question is: Where did this equation come from:
dE/dt=ρ(A.2L)cdT/dt−(A.2L)u′′′
Here is an image:

