Munro85
Marine/Ocean
- May 5, 2022
- 5
Can someone please try and explain this in as simple terms as possible? It is extremely unintuitive to me and I really want to make sense of it in my mind.
I understand how it works in theory but not in reality.
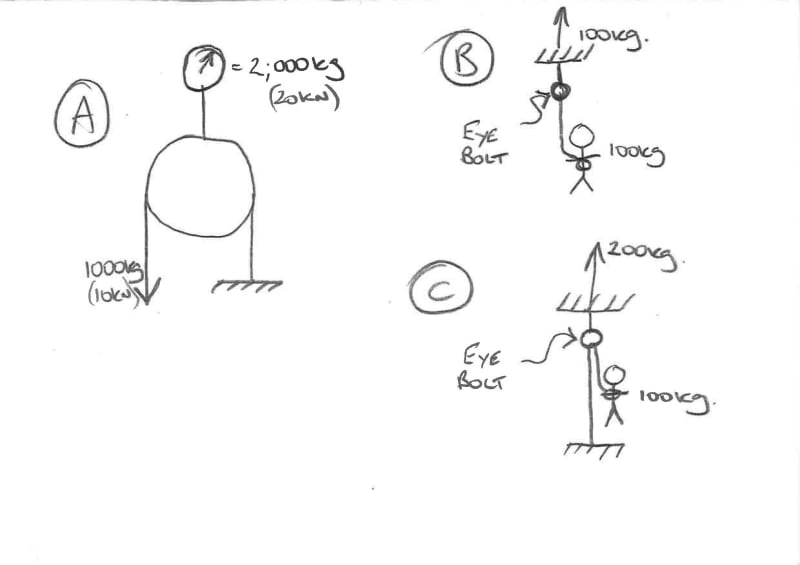
If you look at my little sketch.......Figure (A) shows 1000Kg pulling on a sheave which is attached to the ground.
I can just wrap my head around how the Force that is required to hold the Sheave is 20KN (2,000Kg) I just picture one person pulling on one side with the pull of 1,000Kg equivalent and the same on the other side. These two added together will give the 2,000Kg reading on the load gauge shown.
I then decided to take a very similar example but it was this one that I just couldn't rationalise in my mind.
Image B shows a little person weighing 100Kg. He ties his rope to a little Eye Bolt and straps himself to the rope.
It is clear that if there was a gauge measuring his mass/weight (sorry for using interchangeably) it would read 100Kg.
If however in Figure (C) the stick person fed the rope through the Eye Bolt and secured the end of the rope to the ground, the reading on the Gauge would now read 200Kg.
This stretches my mind too far......Can someone please make some sense of this? Again, from the first example, I can appreciate how it works in theory, but why is it so un obvious in the other example?
Thanks!!
