It’s a common simplifying assumption in linear FEA and structural mechanics in general. You can find the details and equations in classic texts under the term "infinitesimal strain theory". Anyway, small deformation is an assumption that displacements are much smaller than dimensions of the body and thus the undeformed and deformed configurations are identical.

Is this somehow related to using small angle approximation (sin θ ≈ θ)?

When a structure is loaded, it deforms:

x -> x + Δx
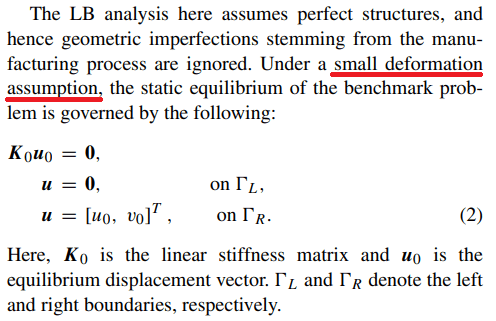
y -> y + Δy
z -> z + Δz

Typically, the applied loads move with the deforming structure.

In linear elastic analysis using small deformation theory, we assume the displacements are much, much smaller than the initial geometry. It is assumed that the displacements are negligible, and we ignore any load / response changes induced by the deformations, such as moment amplification due to the PΔ effect.

E.g. if you apply an arbitrary load set to a simple portal frame, including a vertical load at one of the "knee" joints, that vertical load will not induce a bending moment in the vertical column below the knee. However, if the portal frame sways (as it will under most arbitrary loading conditions), a moment will be generated in the column due to the vertical load multiplied by the column displacement eccentricity. The induced PΔ moment will tend to lead to larger sway displacements than are calculated using small displacement theory.

In small deformation theory, such effects are ignored; in second-order analysis using large displacement theory, the solution is iterated using the structure's displaced geometry until equilibrium is obtained.

http://julianh72.blogspot.com

I haven't rear the previous responses. small deflections means that teh deflected shape is reasonably the same as the original shape. Say you have a simply supported beam length L. under load the length changes to L+dL, but dL << L so equations of equilibrium will use L rather than L+dL.

large displacement models mean displacements are significant. A perfect example of this is a flat plate under pressure. The out-of-plane deflection significantly changes how the structure reacts the pressure loads (from plate bending to in-plane membrane tension)

another day in paradise, or is paradise one day closer ?

A fairly simple example where linear analysis shows its limitations is the "simply supported cable".

If you have a very slender beam, like a wire, and do a linear analysis the deflection will be huge. If you include deformations in the analysis and use supports to include the axial force in the wire, the axial force will carry the wire. The bending contribution will be insignificant.

Thomas