Unique Weld Geometry Evaluation

Question

Unique Weld Geometry Evaluation

tKc74 (Mechanical)

(OP)

12 Feb 21 08:55

Hello, I had posted this along with several other questions here, but I wanted to re-post the weld specific question here as it may get some different insight.

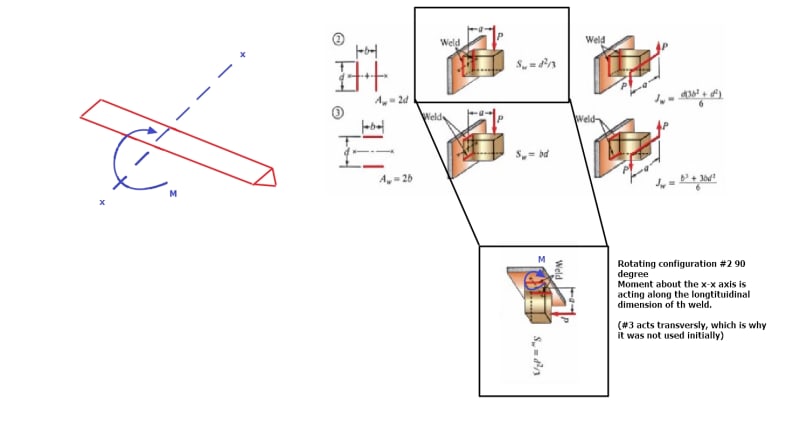
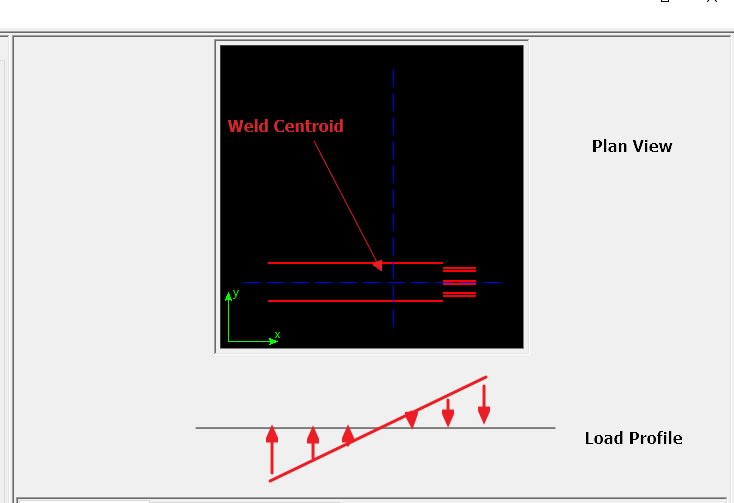
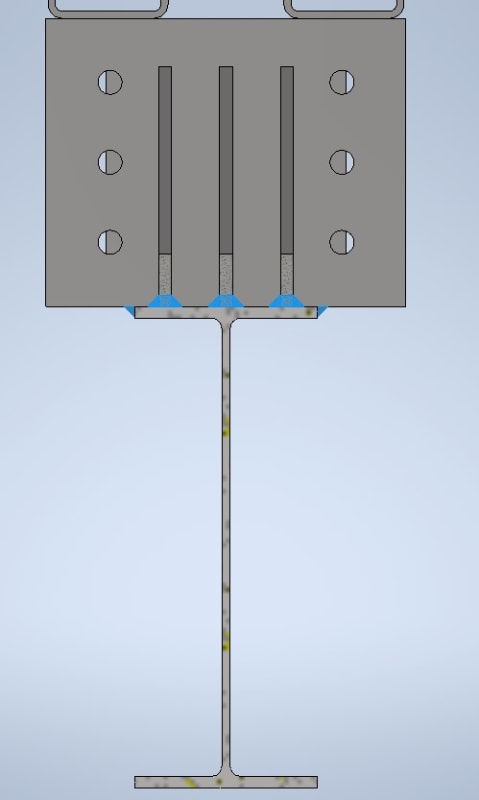
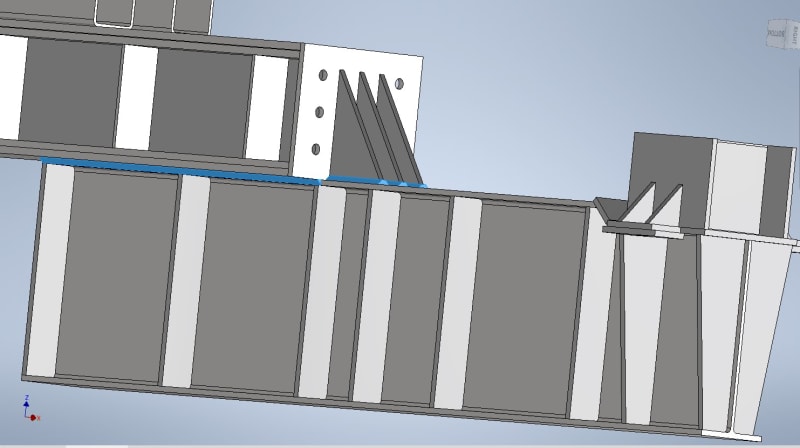
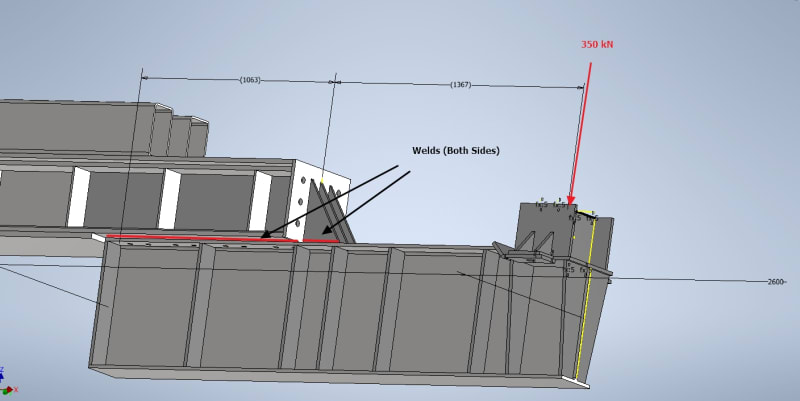
I have a weld and load geometry that I have not been able to find a concrete example on how to evaluate in any textbook or code. I have what is essentially a cantilevered beam cantilevering off another beam, below image best shows the weld geometry with the loads and their dimensions

Originally I attempted to calculate the weld stress conservatively by using #2 above for only the overlapping beam section while ignoring the gussets, calculation as per below:

However this stress is too high, (per AS 3990) need to get to .33σ_ut, which is 142 MPa in my case.

I then went into a deep search on how to add the strength of the gussets to the weld calculation. See Hobert - Welding Formulas and Tables P.17 (P.10 in PDF) to derive any weld shape. Then on P.19 (P.11 in PDF) under 9.3 Example 2 it gives a bit of a worked example of something similar. Following these formulas from these pages I get:
https://files.engineering.com/getfile.aspx?folder=...

Sw_left = 671747 mm2
Sw_left = 1012760 mm2

I then checked against this structural software https://github.com/buddyd16/Structural-Engineering/tree/Python3_migration got the same values (yay!).

I then re-calculate the two (compressive and tensile) stresses in this weld geometry as follow:

My questions/concerns:

(Most Important) Is this a valid weld geometry? Never seen any examples in which a weld is situated in this way relative to its load, I believe its still bending about its neutral axis (y-y), but find it strange I haven't seen any configurations such as this anywhere. It may seem that the load will just overload the first part of the weld it comes into contact with and unzip and not actually rotate about its centroid.
Am I using the right moment arm (Overhang Length + Distance to Centroid) in calculating my stress? or should this be some other distance.
Are there other stress's missing?
The I-Beam and Gusset welds connect to the same object in the same plane, but their weld legs go in opposite directions (up and down), can I still combined the two?

“If the women don't find you handsome, they should at least find you handy.” - Red Green

goutam_freelance (Mechanical) · Answer 1 · 2021-02-12 14:23:48

It is not clear how you selected configuration 2. There should be distribution of weld load on the longitudinal welds in the sense that weld area nearest to P will be high and tensile whereas farthest longitudinal welds may be compressive.There will be high tearing force on welds near load including gusset welds. It is little complex to calculate.

Engineers, think what we have done to the environment !https://www.linkedin.com/in/goutam-das-59743b30/

Tmoose (Mechanical) · Answer 2 · 2021-02-12 17:56:58

By inspection the load paths with the gussets staggered make the vast lengths of the welds on the edges of the poor faying plates fend for themselves.

Are those 7 or 8 bolt holes in the overlap created by the cantilevered beam and the other beam ?

racookpe1978 (Nuclear) · Answer 3 · 2021-02-12 19:03:24

The weld joint is not clear to me either. What is the section view looking through the weld areas?

tKc74 (Mechanical) · Answer 4 · 2021-02-12 23:40:01

Hi Team, thanks for the responses, much appreciated!

I used configuration #2 in original calculation as it seamed more appropriate than #3, #2 has moment applied about the length of the weld (#3 moment is about the weld leg):

However this was replaced with what I calculated based on Hobart and the open source structural analysis software (see OP for links) as the above did not include the gussets:

I agree in that there will likely be a distributed load across the length of the weld, see below, but I need to be able to properly evaluate these stresses with the gussets and that is what I am look for help on.

Quote (goutam_freelance)
There should be distribution of weld load on the longitudinal welds in the sense that weld area nearest to P will be high and tensile whereas farthest longitudinal welds may be compressive.There will be high tearing force on welds near load including gusset welds.

Quote (Tmoose)
By inspection the load paths with the gussets staggered make the vast lengths of the welds on the edges of the poor faying plates fend for themselves.

There are 16 bolts (8 per side) that are designed to take 100% of load separately, but you cannot share bolt and weld loads, lets just focus on weld taking 100% load for now.

See below for a section view (mid way thru the gussets) and another view with welds highlighted as requested:

Thanks in advance!

“If the women don't find you handsome, they should at least find you handy.” - Red Green

dvd (Mechanical) · Answer 5 · 2021-02-13 00:46:21

This thing looks pretty complicated - some background information on this project seems appropriate. There is a bigger picture that is not being shared. Good luck with it.

tKc74 (Mechanical) · Answer 6 · 2021-02-13 00:57:57

Agreed, bit of background information was shared in the link in my OP, but ill copy it here:

Quote (tKc74)
Hello, working on a pair of transport beams to move a large piece of equipment on a flat deck, beams will cantilever off the ends of the trailer to pick up the loads, think Two Unequal Concentrated Loads Unsymmetrically Placed as a load profile. Working to AS/NZ 3990 standard.

Got existing custom beams from a previous move but they are not stiff nor long enough. Increasing stiffness is straight forward enough, adding a plate to the bottom and RHS sections to the top. This also gets deflection down (Δ/l<250).

For length we'll add some UB I-Beams to the bottom of the existing (see below, UB's overhang vary on each end). The UB's top flange can weld to the existing, but thinking belt and suspenders, I will also add some bolts.

Cheers,

“If the women don't find you handsome, they should at least find you handy.” - Red Green

dvd (Mechanical) · Answer 7 · 2021-02-13 01:37:17

Well, you did not ask for help with the big picture, but I ask myself what the cost of bigger, simpler members would be compared with the engineering and fabrication of the proposed.

tKc74 (Mechanical) · Answer 8 · 2021-02-13 01:50:13

Yeah, appreciate the thought, reason why is that the geometry shown above is what it is essentially due to the shape of the equipment being carried and how it is being transported, let’s just say that there are little options other than the geometry already presented, that's why, if possible, I'd like to keep the topic of conversation on how to evaluate the above weld.

“If the women don't find you handsome, they should at least find you handy.” - Red Green

kingnero (Mechanical) · Answer 9 · 2021-02-13 09:01:40

Basically, sigma = M/W + F/A
with M = overhang + distance of outer edge of welds to centroid of welds, and W of the welds, as you already mentioned.
and I'd somehow limit the A (to the first 50% ?) because I can't see the vertical force being transferred all the way to the end.

goutam_freelance (Mechanical) · Answer 10 · 2021-02-13 12:28:11

The complexity arises as the integrity of a single beam is broken. The source of uncertainties are the following:
1. The exact distribution of loads on longitudinal welds is not known
2. The stress concentration factor on most vulnerable welds can not be calculated exactly.
3. Welds in tension are notoriously weak against fatigue.

To reduce the uncertainties I feel that the welds at most vulnerable part (beginning of junction area of two beams) should not be subjected to tension. So the alternative proposal is as follows:

The formed plate(hot formed) to be sized suitably for the load. All existing arrangements can be kept for double safety.
The advantages are as follows:
1. The tension load on critical welds is mostly removed.
2. Member to member load transmission is mostly by compression. The plate is subject to tension
3. The stress on top beam web which has highest stress is reduced.

Engineers, think what we have done to the environment !https://www.linkedin.com/in/goutam-das-59743b30/

Come Join Us!

Posting Guidelines

Unique Weld Geometry Evaluation