Mateus_R
Mechanical
- Mar 15, 2018
- 48
Dear all,
I have to evaluate the effect of the buckling in a bar, and some questions arise, specially regarding the ANSYS Mechanical interpretation:
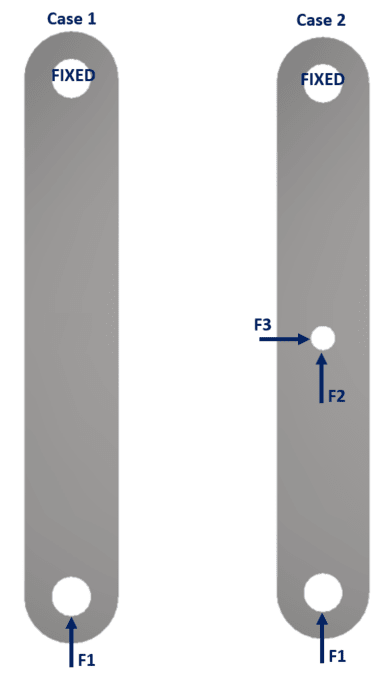
Suppose I evaluate buckling according to image attached, case 1 -> Ansys will give me the critical buckling load related to the applied load, by means of the loading factor, and this is straightforward.
Suppose case II - now I have two compression forces located at different points. Ansys still gives me the loading factor, but in this case how can I interpret it? How the loading factor is related to each of the two forces?
Thank you for your support.
I have to evaluate the effect of the buckling in a bar, and some questions arise, specially regarding the ANSYS Mechanical interpretation:
Suppose I evaluate buckling according to image attached, case 1 -> Ansys will give me the critical buckling load related to the applied load, by means of the loading factor, and this is straightforward.
Suppose case II - now I have two compression forces located at different points. Ansys still gives me the loading factor, but in this case how can I interpret it? How the loading factor is related to each of the two forces?

Thank you for your support.
