Existing Post tension beam doesn't meet what the existing loads would require 4

jplay2519 · Oct 20, 2015

I have an existing structure I'm analyzing and I'm just not matching something. It's a parking deck, live load = 40 psf. Beam is 20" wide x 26" deep, pan joist system is 6" side x 18" deep with 4" slab (6" x 14" + 4" slab). The Beam span is 57' and the tributary width is 28'. The effective prestress force is 350 kips. At the center of the beam positive auxiliary rebar consists of 2#5 and 2#6 bars. At the support negative auxiliary rebar consists of 3#7 and 2#8 bars. I'm getting a capacity of 712 k-ft positive and 872 k-ft negative. I am getting a DEMAND of 630 k-ft positive and 1262 k-ft negative. This can't be right. I'm just checking the existing loads, I haven't put the proposed loads or anything on there. d=23.75". I'm not sure what I'm missing, I may be burnt out and missing something to simple that I may be booted from the forum but any help would be greatly appreciated.

KootK · Oct 20, 2015

Is the load balancing effect due to tendon drape properly accounted for?

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

jplay2519 · Oct 21, 2015

No. What's the general procedure for that or do you have a reference I could run through.

KootK · Oct 21, 2015

Well, lucky you then. It's not every day that you get your engi-world rocked: Link. It still feels a bit like cheating to me at times.

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

hokie66 · Oct 21, 2015

Just to clarify, the ultimate flexural strength check is quite separate from load balancing. That said, I think most slabs with appropriate load balancing for serviceability will also be adequate in ultimate strength.

Ingenuity · Oct 21, 2015

As hokie66 correctly states, ultimate flexure has nothing to do with 'load balancing'.

jplay2519 said:
DEMAND of 630 k-ft positive and 1262 k-ft negative

Are you assuming wL²/24 for M⁺ and wL²/12 for M^-?

Doible check your effective prestress of 350 kips (or 14 x 1/2" dia tendons).

Assuming this is an internal span, with maximum drape (h = 21.5"), the equivalent balanced load is approx 1.54 klf [from P x h = wL²_bal / 8 ]

Assuming a pan joist spacing of 30", your equivalent slab thickness is 6.8" over the trib width, so including the SW of the 26" x 20" beam the total applied SW is approx 2.75 klf.

Only load balancing 56% of SW which is low...so maybe recheck your P_eff from the existing design or shop drawings.

Of course, if my assumption of the pan joist spacing of 30" is wrong, so too is my calculated balanced load

jplay2519 · Oct 21, 2015

The pan joist spacing is 66 actually, sorry for leaving that out. Thanks for the link I'll check that out and see what I can figure out.I'm at the end span of the building so its a discontinuous end with the integral support. Thanks for the support guys.

jplay2519 · Oct 21, 2015

Oh and I am assuming wl^2/24 and wl^2/12.

jplay2519 · Oct 21, 2015

The drawings definitely say 350k effective prestress. I'm at a loss because the negative demand is about double what the capacity is and I've been through that example KootK sent (which is a gem thanks man). It amount of balance seems low and the moment capacity is definitely off. I guess I'll scan in the sheets for a last shot at you guys taking a look.

KootK · Oct 21, 2015

@jplay: I played the odds and assumed this to be a north american unbonded PT beam. Is that the case?

@Hokie/IDS: Your statements imply that there was something inaccurate or misleading in mine. And I dispute that. I believe that you're simply viewing things from what I have come to see as the "Aussie perspective" on PT design. And that is likely influenced by the prevalent use of bonded PT in your area.

hokie66 said:
Just to clarify, the ultimate flexural strength check is quite separate from load balancing.

IDS said:
As hokie66 correctly states, ultimate flexure has nothing to do with 'load balancing'.

From a design perspective, one can consider the benefit of PT drape in at least two ways:

1) As an internal action improving sectional resistance or;
2) As an external action balancing the applied loads (Link).

Either way, PT drape absolutely does alter the moment Demand/Capacity situation. As such, it's entirely plausible that one -- particularly a north american -- might have neglected to include the benefit of PT drape along with potentially eccentric pre-compression.

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

jplay2519 · Oct 21, 2015

Here are the plans and associated details/schedule. I'm looking at the beam on G19, between GD and GE. Tendons are unbonded, from the notes on the drawings is the only way I got this. I was assuming unbonded as well though before I saw that.

JAE · Oct 21, 2015

Hmmm...All my hand calculations for PT beams (bonded or unbonded) for ultimate strength didn't get affected by load balancing...only the internal concrete stress checks at the various stages.

The ultimate strength only depended on the f'c, the fy of the reinforcement, the locations and areas of strands and mild rebar, and the overall geometry of the beam itself.

The demand (required moment capacity) did get affected by the secondary moments in continuous members due to the prestress force and strand location. Maybe that's what KootK is referring to.

Check out Eng-Tips Forum's Policies here:
faq731-376

KootK · Oct 21, 2015

JAE said:
The demand (required moment capacity) did get affected by the secondary moments in continuous members due to the prestress force and strand location. Maybe that's what KootK is referring to.

Certainly, that is one way to think of it. I wouldn't consider the effect secondary, however, as I'm used to that term being associated with the less significant hyperstatic effects.

All I really stated initially is that PT drape helps resists load and should be accounted for in some fashion, which is obvious. Otherwise, we wouldn't have the Golden Gate Bridge to admire. Whether you view the effect as an externally applied balancing load, or a boost to ultimate member capacity, is immaterial and simply a matter of choice.

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

jplay2519 · Oct 21, 2015

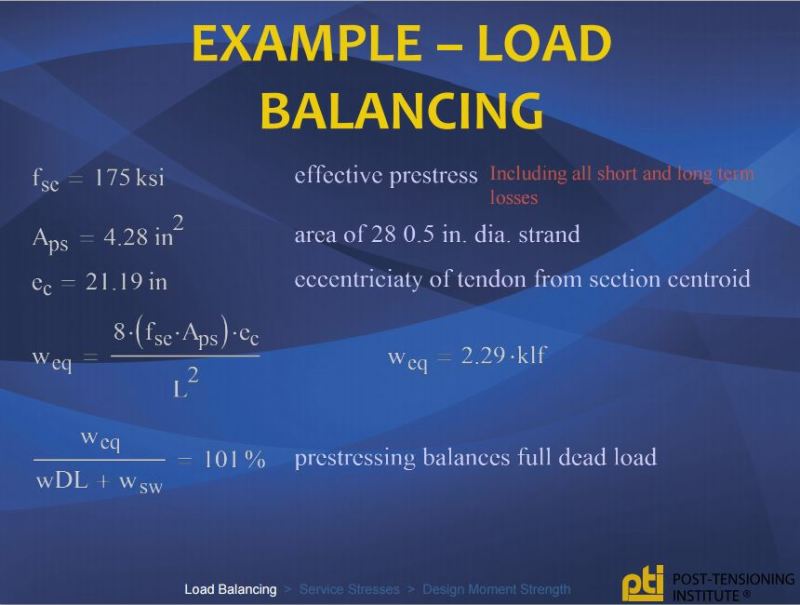
It seems in the PTI presentation that they still use all of the load for Mu (dead plus live) when the dead load was shown to be balanced out (page 43). The capacity of the beam is checked against this number, so I don't see how the balancing helped out in capacity or demand in that example. I understand the concept of incorporating the drape and it makes sense, I remember discussing in advanced concrete a little bit. I'm not sure how to actively use it though, because even if I update the stresses the Mn vs Mu is still way out of wack.

KootK · Oct 21, 2015

Can you direct me to that example/publication jplay? It would be great to have a common basis for discussion.

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

jplay2519 · Oct 21, 2015

Oh I'm referencing the link you sent earlier. Link. The section 3 of a series it seems.

hokie66 · Oct 21, 2015

KootK,
The ultimate strength at a given section depends only on the reinforcement (of both kinds), its location, and the concrete. Whether it is draped or not does not affect the ultimate flexural strength.

jplay2519 · Oct 21, 2015

Leave it to a VT guy to crush my hopes and dreams lol. I'm a Clemson Alum

. Not sure I should be saying that considering my ignorance of PT.

mike20793 · Oct 21, 2015

I highly recommend the book "Post-Tensioned Concrete: Principles and Practice" by Dirk Bondy and Bryan Allred. Dirk's father, Ken, is one of the pioneers of PT design in North America and this is an incredible PT resource from basic concepts all the way to common field errors and practical detailing for constructability. You will be hard pressed to find a better reference for PT design. The chapter on flexure is very straight forward and does an excellent job explaining ultimate strength of indeterminate systems. I can probably scan a few pages and include them later if you're still having trouble, jplay.

If anyone ever gets a chance to view his PT class from UCLA, you will not be disappointed. His commentary on the confusing shear provisions in the code and secondary effects in indeterminate systems alone makes it worthwhile. His father, Ken, is a "Teacher's Assistant" so you get good info from people that have been doing it for over 60 years combined.

KootK · Oct 21, 2015

@Mike: I picked up the Bondy book the last time that you shamelessly plugged it. It's everything that you promised and then some. The only disappointment was that it showed up at my door as soft cover! An inglorious fate for such a fine piece of work. I was tempted to run it to our printer and have it hard bound myself.

jplay said:
It seems in the PTI presentation that they still use all of the load for Mu (dead plus live) when the dead load was shown to be balanced out (page 43).

Not so fast. I believe that w_0p represents the effective balancing load and would in fact be negative. I've KootK-ized the example below to show how PT drape effects the bottom line when it comes to bending capacity/demand. I think that you'll get the idea.

I like to debate structural engineering theory -- a lot. If I challenge you on something, know that I'm doing so because I respect your opinion enough to either change it or adopt it.

Eng-Tips is the largest engineering community on the Internet

Intelligent Work Forums for Engineering Professionals

Existing Post tension beam doesn't meet what the existing loads would require 4

Structural

Recommended for you

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Structural

Similar threads

Log in

Part and Inventory Search

Sponsor