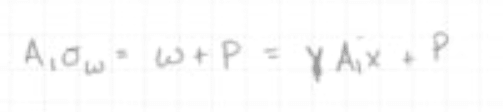Hey All,
I'm revisiting classical stress analysis and working through Strength of Materials, Part 1 (3rd ed.) by Timoshenko. I'm currently stuck on problem 8, particularly with deriving equation (a). While the equation intuitively makes sense—where the change in area times the working stress is proportional to the weight of the change in volume—I'm having trouble proving it mathematically.
I've attached scans of the problem and two of my attempts at the derivation. Any insights or guidance on how to approach the derivation of equation (a) would be greatly appreciated! As of right now, I'm my best guess is my Answer 1 is correct and dA*dx is so small it goes to zero.
** Also clarifying I'm not a student asking for homework/project solutions. I'm not in school, just trying to improve as a structural analyst **
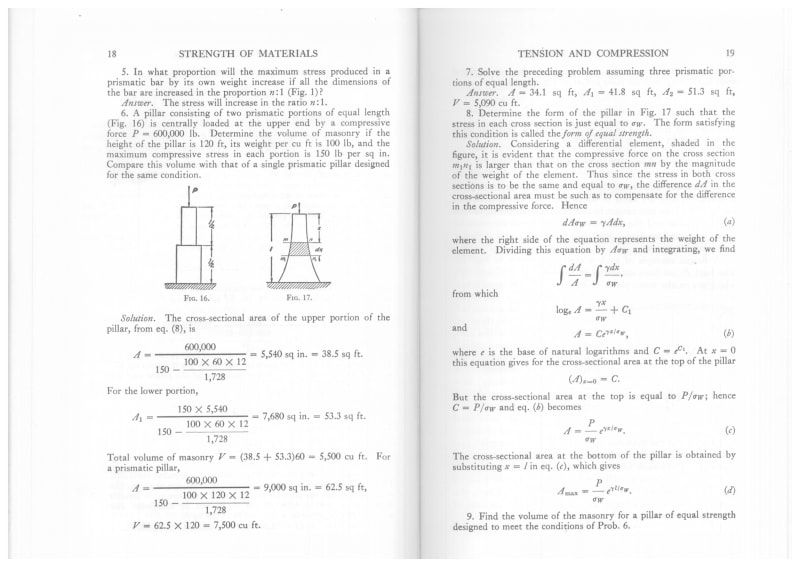
Determine the form of the pillar in Fig. 17 such that the stress in each cross section is just equal to working stress. The form satisfying this condition is called the form of equal strength.
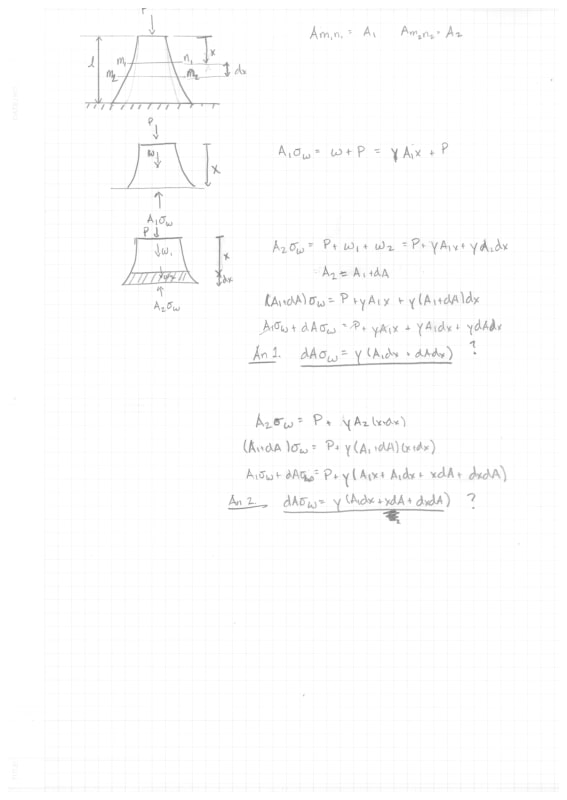
I'm revisiting classical stress analysis and working through Strength of Materials, Part 1 (3rd ed.) by Timoshenko. I'm currently stuck on problem 8, particularly with deriving equation (a). While the equation intuitively makes sense—where the change in area times the working stress is proportional to the weight of the change in volume—I'm having trouble proving it mathematically.
I've attached scans of the problem and two of my attempts at the derivation. Any insights or guidance on how to approach the derivation of equation (a) would be greatly appreciated! As of right now, I'm my best guess is my Answer 1 is correct and dA*dx is so small it goes to zero.
** Also clarifying I'm not a student asking for homework/project solutions. I'm not in school, just trying to improve as a structural analyst **
Determine the form of the pillar in Fig. 17 such that the stress in each cross section is just equal to working stress. The form satisfying this condition is called the form of equal strength.