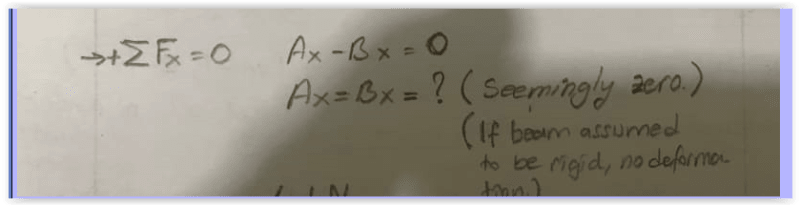Stability Of Simple Supported Beams With An Internal Hinge 5

[CaptHope]

Hi dear friends.I was reviewing and remembering very basic concepts of structural analysis course for my MSc entering exam since it had been 16 years after my graduate. While I was solving some problems on stability and determinacy of structures, I encountered a truss problem. While solving that one, I was trying to analyze the configuration of its members to try to figure out its internal stability visually. Then I failed, my answer, which was relied upon try to figure out the movement possibilities of joints using geometrical properties of them, was wrong. And then I started to try to understand what I did/thought wrong. So, at the end, the below problem has arised as a sample of my thinking way during this problem.

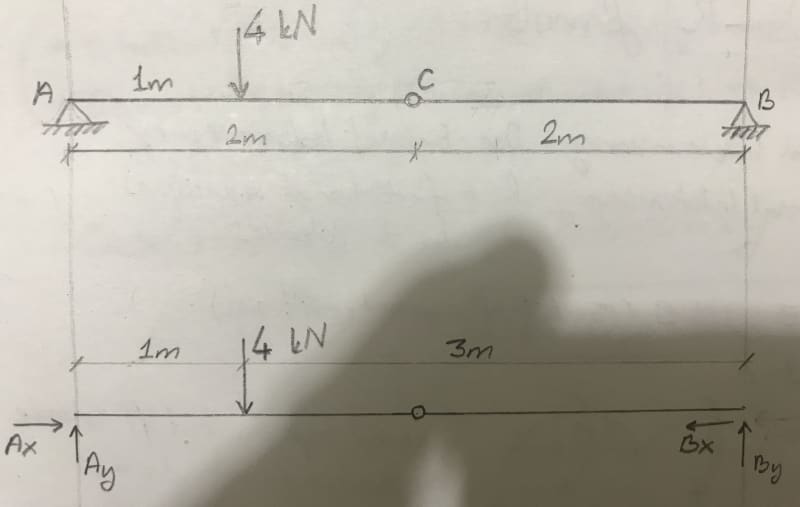
It is a simple supported beam at two ends with an internal hinge.

If you write equilibrium equations:

Then, for the section that is cut trough the hinge:

Since the equilibrium can never be satisfied in this way, I say it is not stable.

But, the below is another approach using geometry:

And this time, since it is impossible to move point C downwards without deforming the rods, and since the rods assumed to be rigid, then I conclude that this beam is stable.

My mind is mixed up a bit. But I think I may be overlooking some very basic assumptions about statics, structural analysis, etc.

Can you help me figuring out what I am overlooking or where I am wrong?

Thank you.

 

[rb1957]

ok, sigh. but stress and strain are co-dependent. A material fails because it reaches ultimate strength and ultimate strain.
I have trouble seeing how a material could have adequate strength but fail by strain ?
unless you're applying a service-ability constraint (and limiting deflection).
But then it isn't the material failing, but the design (having reached, intact, a design constraint).

another day in paradise, or is paradise one day closer ?

 

[BAretired]

if one end is a roller, then the beam cannot develop the required axial loads and would collapse ...
I guess you could call that "instability" or a "mechanism" ... I'd call it "broken".

A beam with pin/roller support is stable and determinate. Adding a hinge in the span makes it unstable, not broken.

Aren't "strength" and "strain" interchangeable (due to Hooke's law) ?

No! Hooke's Law says that for an elastic material, strain is proportional to stress, but strength is also proportional to cross sectional area as well as stress. A beam with small area could fail due to excessive stress, irrespective of the magnitude of the deflection.

BA

 

[BAretired]

I have trouble seeing how a material could have adequate strength but fail by strain ?

It would not fail by strain. You stated "We seem to be showing that deflection of the beam is limited by the strength of the material". It would be more accurate to say that "We seem to be showing that deflection of the beam is limited by the strain of the material.

BA

 

[CaptHope]

Dear friends,
I am glad that my post causes quality technical discussions. Thank you all for your valuable responses. I will soon add some other problem related to/similar this one but about trusses. And I think it will help me to explain why I came up with this kind of a problem.
For discussions on stress-strain, I don’t think stress-strain relations, and hence strength issues are of primary importance for this problem. As far as remember, when we study on stability and determinacy of structures, we don’t care about what material is used, what’s the moment of inertia of the sections, etc. The parameters of interest are number of support reactions, number of members, number of joints, configuration of supports and configuration of members. So, I think assuming members as rigid bodies on which no deformation can occur should not be a wrong approach. It seems to me as if this problem is about assumptions made in the very beginning of structural analysis subject/course rather than what really happens to the real physical structure.

Umit KARAHAN
Civil Engineer

 

[Agent666]

So, I think assuming members as rigid bodies on which no deformation can occur should not be a wrong approach.

In this particular case it does matter because the vertical deflection at C, and hence load developed horizontally at the supports is primarily dependant on the axial stiffness of the member selected. If you made it axially rigid then it can't deflect due to the rigid body constraints, hardly realistic.

 

[rb1957]

three statement in your original post are incorrect.

Ax and Bx are equal and opposite, and non-zero.
You should have realised that CB is a two force member and therefore the reaction is aligned to the member axis.

This should have been a clue that something is wrong.

This too is wrong, and contradictory to the previous statement (which is probably why you posted here).
The assumption that members cannot deform (elongate) is incorrect. With this assumption no structure can work.
Maybe this is a mis-remembered assumption ... typical assumption is "small displacements" where the displacements don't affect the structure.
This problem is an example of "large displacements" where the deflection of C is required to make the structure work.

I think we've shown (like agent above) that C deflects down. I've shown that this displacement cannot be determined from equations of equilibrium, as this is an indeterminate structure. This needs some other equation to solve ... likely contenders are energy equilibrium (the potential energy of the load compared to the strain energy of the structure) or compatibility of the strain of BC compared with the geometrical change in length due to the deflection of C.

another day in paradise, or is paradise one day closer ?

 

[dauwerda]

It seems to me that the OP is confused about the very premise of what it means for a beam to be simply supported. As others have already mentioned, the definition of a simply supported beam is a beam with a pin support on one end and a roller support at the other.

As far as remember, when we study on stability and determinacy of structures, we don’t care about what material is used, what’s the moment of inertia of the sections, etc. The parameters of interest are number of support reactions, number of members, number of joints, configuration of supports and configuration of members. So, I think assuming members as rigid bodies on which no deformation can occur should not be a wrong approach. It seems to me as if this problem is about assumptions made in the very beginning of structural analysis subject/course rather than what really happens to the real physical structure.

I get the impression the OP is expecting to solve for a simply supported beam (as is stated more than once) but has forgotten that one of the supports needs to be a roller, and has quickly moved out of the realm of simply supported, statically determinate structures. As shown in the FBD, with two pin supports, it is a statically indeterminate structure and this does require the use of material properties to solve for reactions/equilibrium.

If one of the supports is changed to a roller support (as the title of the thread implies it should be), it will make this a unstable structure.

 

[rb1957]

yes, but he did draw both supports as pins, neither as a roller. And yes, typically a SS beam has a roller end (to remove axial load redundancy). But this is a typical assumption to remove an analytical inconvenience; in the real world we don't (typically) build beams with roller supports ... sometimes we do carefully release this constraint.

But as you say, if a roller, then no axial load, then collapse (or unstable).

another day in paradise, or is paradise one day closer ?

 

[CaptHope]

Sorry for my mistake of mis-naming the supports. The supports are as I drawed. They are pin supports. Only moment free.

Umit KARAHAN
Civil Engineer

 

[dauwerda]

The supports are as I drawed. They are pin supports. Only moment free.

If that is the case, this is a stable, but statically indeterminate structure. Analyzing it does require the use of material properties, you can read a bit more about that here:

https://en.wikipedia.org/wiki/Statically_indeterminate

 

[CaptHope]

f that is the case, this is a stable, but statically indeterminate structure. Analyzing it does require the use of material properties, you can read a bit more about that here:

https://en.wikipedia.org/wiki/Statically_indetermi...

Yes, I know it. If the number of unknowns are greater than known static equilibrium equations, 3 for planar and 6 for space structures, then the structure is statically indeterminate and you need additional equations which are derived from load-deformation relations. After all, I am a civil engineer, not someone who is an engineer enthusiast and tries to learn from google.
The problem I mentioned points something related to made assumptions/definitions. I am quite aware of what is the case for this structure in real conditions, such as the impossibility of preserving its shape unless there occurs some axial forces, impossibility of pre-defined rigidity in real life etc.
My question is, if that kind of a problem is given you, for example in an exam, what would you say? a) It is stable. b) It is unstable. c) The question is wrong, it has some conceptual contradiction.
Anyway, thank you for your reply and additional link for more information.


Umit KARAHAN
Civil Engineer

 

[rb1957]

d) none of the above

If this was the complete question, as sketched in the OP, then
it could be stable (or unstable) depending on the strength (or strain) of the members.

another day in paradise, or is paradise one day closer ?

 

[Earth314159]

My question is, if that kind of a problem is given you, for example in an exam, what would you say? a) It is stable. b) It is unstable. c) The question is wrong, it has some conceptual contradiction.

It is stable.

It is a second order structure. It relies on deformation to be stable. Linear elastic analysis of determinant or indeterminant structures assumes that small deformations can be ignored. This basic assumption is not valid in this case.

It is indeterminant by definition since the basic equation of equilibrium by themselves can not be used to solve the load path. However, it is not redundant. You need the axial stiffness EA/L to determine the deflections and the internal forces.

You need account for the stiffness of the structures that rely on deformations for stability.

If you argue that the strength can govern, that is true of any structure that you consider for stability. The basic assumption regarding stability is that the structure is strong enough. Strength is a separate issue. Otherwise, you would always have to qualify that the strength does not govern when checking for stability.