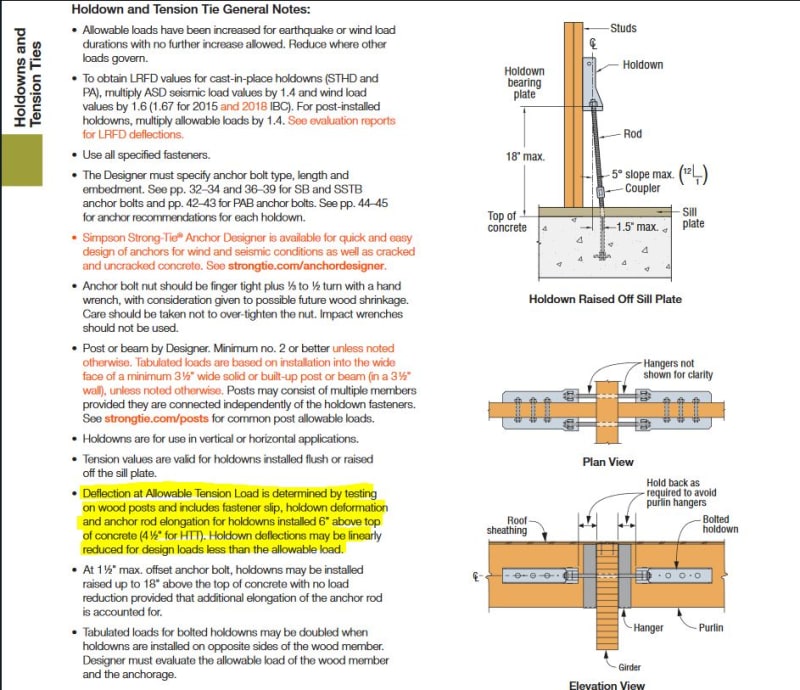
I'm taking a close look at some of my shear wall calculations and deflection compatibility between co-linear shear walls. The hardest thing for me to be happy with is the deflection of the panel caused by hold down elongation/slip.
Simpson publishes (both in their catalog and also in the third party reports) vertical displacement of the anchorage due to elongation and slip at maximum allowable load. I realize this value is non-linear, but is there anything out there that could be used to approximate this value with some accuracy at load levels below max allowable? I don't generally design my shear walls to hit allowables exactly, and like any self respecting, OCD engineer, I can't stand that I'm using a max value in a less than max situation.
I can see some value in assuming that it just maxes out on that piece of the deflection puzzle (and, indeed, this is how I typically handle it), but it's just not...precise.
How are others treating this term in the deflection equation? Is this a valid concern, or am I trying to round to the thousandths with inputs truncated at multiples of a hundred?
Simpson publishes (both in their catalog and also in the third party reports) vertical displacement of the anchorage due to elongation and slip at maximum allowable load. I realize this value is non-linear, but is there anything out there that could be used to approximate this value with some accuracy at load levels below max allowable? I don't generally design my shear walls to hit allowables exactly, and like any self respecting, OCD engineer, I can't stand that I'm using a max value in a less than max situation.
I can see some value in assuming that it just maxes out on that piece of the deflection puzzle (and, indeed, this is how I typically handle it), but it's just not...precise.
How are others treating this term in the deflection equation? Is this a valid concern, or am I trying to round to the thousandths with inputs truncated at multiples of a hundred?