-
1
- #1
cal91
Structural
- Apr 18, 2016
- 294
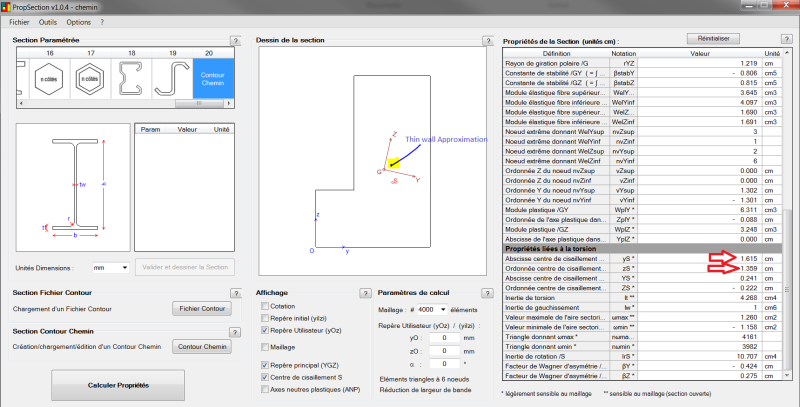
In the NCEES Practice Exam I was reviewing this problem, where the torsion due to the reaction is asked for. The solution calculated the eccentricity with respect to the center of gravity.
I would've thought that the eccentricity would be with respect to the shear center...
For solid sections, is the shear center the center of gravity? If not, how do you calculate it?
All of the resources I have (text books, internet) only provide information for thin wall sections, not solid sections for calculating shear centers. Does anyone know of a source for solid sections?
Thanks
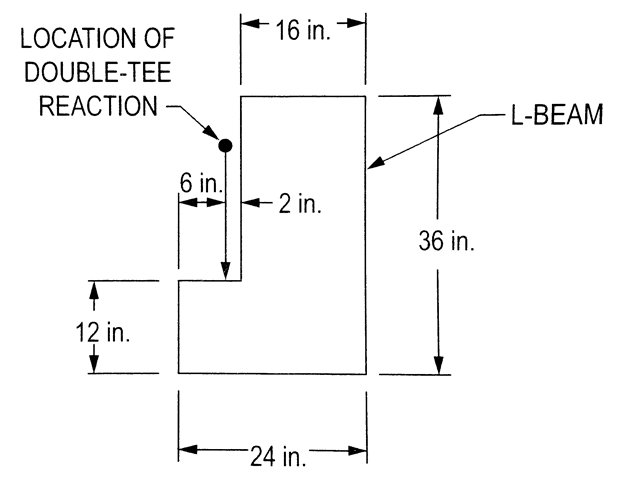
I would've thought that the eccentricity would be with respect to the shear center...
For solid sections, is the shear center the center of gravity? If not, how do you calculate it?
All of the resources I have (text books, internet) only provide information for thin wall sections, not solid sections for calculating shear centers. Does anyone know of a source for solid sections?
Thanks