Fluid Temperature Rise Due to Net (Insolation + Radiation Loss + Convection Loss)

[Alan_VA]

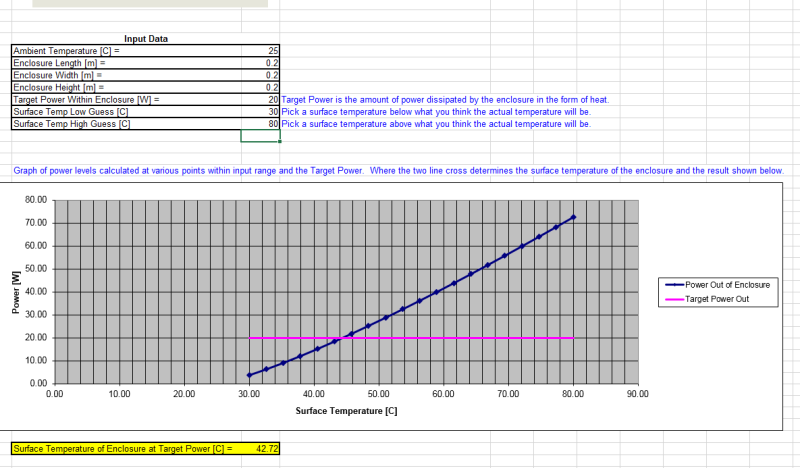
I have a problem which should be fairly simple, but which is beating me up pretty severely. Situation: A static water-filled insulated pipe sitting in the sun. I'm trying to determine the change in water temperature when subjected to a known cycle of air temperature and solar radiation. I have read thru several threads on similar topics here at Eng-Tips. The problem with the prior solutions is that all presume that the temperature of the water is a known value; very similar to most heat transfer problems that are concerned with calculating the heat flow required to maintain a given environmental condition.

This problem has two unknowns, water temperature (Tw) and temperature of the insulation outer surface (To), with Tair, the insolation, and the physical properties known. I can't figure out how to build another relation between Tw and To. Thoughts?

Alan

 

[hendersdc]

For this kind of problem you need to just pick a water temperature, find the resulting outer surface temperature, and then see if the total heat transfer adds up with the chosen temps and is in equilibrium or not. If not change your temperature guess and try again. You will need to make assumptions like uniform temperature distribution around the circumference and throughout the water in the pipe to make the calculations easier.

I have created spreadsheets for similar problems (a box of unknown surface temperature but know dimensions and power output with only natural convective heat loss) in the past that cast a wide net on temperature guesses and then interpolate the results to find the correct value.

If you don't want to automate it so much just put all the formulas together in a spreadsheet and keep changing the input temperature until your heat out and heat in match.

Here is a screenshot of the example I talked about, I use this as a quick first order approximation to determine if power dissipation within a device is going to be an issue or not:

 

[Alan_VA]

Thank you for the input. I was already leaning toward a "goal seek" sort of solution. Please correct me if I'm over-complicating the problem, but, since there are two unknowns with only one equation, why wouldn't I end up with a family of answers, rather than a single unique (Tw, To) pair?

Alan

 

[hendersdc]

I am assuming you can find equations to tie To and Tw together so that guessing one will give you a value for the other. This may not be the case. Things that will complicate your calculations will be any heat transfer to water/pipe further down the line that is not exposed to the sun. If not then you may need to specify a grid of (Tw,To) values instead and try and find a best fit value based on that. In that case I would think there would be two sets of valid solutions (one with Tw>To and one with To>Tw) and you will have to pick which one is most likely to be the case.

 

[Latexman]

On problems like this, one must use their engineering judgment to "bracket" the starting water temperature. hendersdc is spot on. If the design cannot handle the minimum or maximum starting water temperature, then, it's back to the drawing boards!

Good luck,
Latexman

To a ChE, the glass is always full - 1/2 air and 1/2 water.

 

[IRstuff]

Depends on what you are trying to solve for. It's actually quite a transient problem, and depending on the volume and surface area of the pipe, it might be quite laggy in temperature response.

One thing to do first is to calculate the Biot number and determine whether a simple lumped analysis will get you there. If not, the you'd need to do a finite element solution.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[Alan_VA]

In the geometry of the problem the Biot number will be in the range of 15-20, so a transient problem it is. Oh, joy.

I found three papers on the subject:
"Transient analytical solution to heat conduction in composite circular cylinder", Lu, et al, Int'l J of Heat & Mass Transfer, 49, 2006, 341-348
"Analytical solution to transient heat conduction in polar coordinates with multiple layers in radial direction", Singh, et al, Int'l J of Thermal Sciences, 47, 2008, 261-273
"Heat conduction problem in a two-layered hollow cylinder by using the Green's function method", Kula and Siedlecka, J of Applied Mathematics and Computational Mechanics, 2013, 12(2), 45-50

In all three papers, the math looks like pretty hard slogging for a guy whose last calculus class was 35 years ago. Any suggestion on an FEA modeling tool instead?

 

[IRstuff]

Well, FEA in the sense that you need to discretize the problem, so even Excel or Matlab might be doable.

It's possible you could fudge it into a 1D transient analysis and modify the few choices available in the file exchange:

http://www.mathworks.com/matlabcentral/fileexchange/?utf8=✓&term=transient+heat+transfer

There appear to be a couple of possibilities in Excel:

https://www.google.com/url?sa=t&rct...WzOf1tN3QAhVmJJoKHYmPA0YQFggrMAM&url=https://

http://www.asee.org/public/conferen...hjD0dVuBvPB9tG2uZZjCKQ&bvm=bv.139782543,d.bGs

http://homeenergypros.lbl.gov/group...ent-heat-transfer-analysis?xg_source=activity

The latter seems to be configurationally like your problem

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[Alan_VA]

Thanks for the suggestions.

 

[IRstuff]

I vaguely recall a wonderful thing called a Schwartz-Christoffel transform from AMa95, which would allow one to map shapes into other, more useful shapes, like a circle into a half-plane, but, AMa95 was about 40 yrs ago...

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm