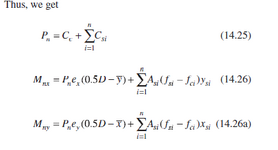Column Design - concrete - Biaxial bending - Why the r/f area is not deducted from concrete area

[Pretty Girl]

I'm trying to understand the concept behind this. This is from "Reinforced concrete design to eurocodes" by prab bhat, page 370.
I'm bit confused of why the reinforcement area is not deducted from the compressed area of the concrete, when it calculates the "axial force in concrete (Nc)".

If we assume that it considers the r/f area as concrete (because r/f is more than sufficient to substitute concrete), still the calculation added compressive r/f area in the "axial force in steel (Ns)".

So it's like double adding the r/f area into the compressive steel area and also to the compressive concrete area (since the r/f area doesn't get deducted from concrete area).

Isn't it inaccurate to not to reduce r/f area from compressive steel if we already added that in compressive r/f area calculation?

Does that mean the calculation in the book not that accurate?

 

[Retrograde]

Because the area of reinforcement is probably only a few percent of the total area of the column, deducting the area of the reinforcement will make little difference to the answer.