Tek-Tips is the largest IT community on the Internet today!
Members share and learn making Tek-Tips Forums the best source of peer-reviewed technical information on the Internet!
-
Congratulations TugboatEng on being selected by the Eng-Tips community for having the most helpful posts in the forums last week. Way to Go!
Actual Liquid Velocity Calculation
- Thread starter ChihebG
- Start date
LittleInch
Petroleum
With difficulty.
First you need to know the flow regime and second the actual volume fraction in the pipe at the point where you are looking at. Both normally come from the transient flow analysis tool you are using. They may also give you the answer directly.
Slug flow or very wavy flow, the actual velocity will vary wildly and change constantly.
Stratified and wavy in a flat pipe you might get less variation, everything else, huge variation.
Why do you need to know?
Remember - More details = better answers
Also: If you get a response it's polite to respond to it.
First you need to know the flow regime and second the actual volume fraction in the pipe at the point where you are looking at. Both normally come from the transient flow analysis tool you are using. They may also give you the answer directly.
Slug flow or very wavy flow, the actual velocity will vary wildly and change constantly.
Stratified and wavy in a flat pipe you might get less variation, everything else, huge variation.
Why do you need to know?
Remember - More details = better answers
Also: If you get a response it's polite to respond to it.
- Thread starter
- #3
EmmanuelTop
Chemical
For a known liquid flowrate (m3/hr) and volume fraction (m3 liquid / m3 gas+liquid) the calculation is straightforward. It does not change with the flow regime because liquid is incompressible fluid and it will always occupy the same percentage of the cross-sectional area of the line, that is required to pass given volume of liquid in unit of time. The catch is not to know the flow regime, but to know the actual flowrate - if you know the flowrate then you have the velocity.
This is all great on paper, but the reality is somewhat more complex. If you can place a bet that your actual liquid flowrate in any section of the line is as someone claims to be, then the calculation is again simple and straightforward. More often, you will find that actual velocities will vary so much along the line that it is impossible to confirm the actual velocity/flowrate unless you actually measure it. Gas and Liquid move with different velocities. Slugging is one consequence of this phenomenon. Depending on many parameters, your actual velocity can be anywhere between zero and the velocity at fully dispersed flow.
Dejan IVANOVIC
Process Engineer, MSChE
This is all great on paper, but the reality is somewhat more complex. If you can place a bet that your actual liquid flowrate in any section of the line is as someone claims to be, then the calculation is again simple and straightforward. More often, you will find that actual velocities will vary so much along the line that it is impossible to confirm the actual velocity/flowrate unless you actually measure it. Gas and Liquid move with different velocities. Slugging is one consequence of this phenomenon. Depending on many parameters, your actual velocity can be anywhere between zero and the velocity at fully dispersed flow.
Dejan IVANOVIC
Process Engineer, MSChE
Here is a page from my 5-day course, that (I hope) shows the difficulties that Dejon is talking about. The mass flow rate is identical in every segment. One joint down in the no-BackPressure (left) side, the gas takes a lot of room so the liquid really has to scurry to keep the mass flow rate constant. At the bottom of the well, the hydrostatic head has squashed the gas into a smaller space and the liquid doesn't have to go as fast. The 2 photographs are all of the same water flow and all have about the same mass (and volume) flow rate.
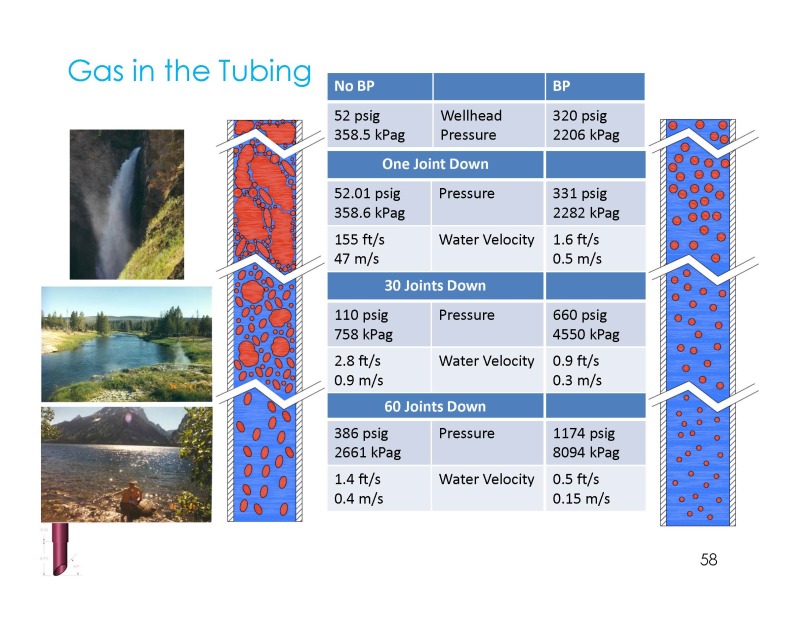
David Simpson, PE
MuleShoe Engineering
In questions of science, the authority of a thousand is not worth the humble reasoning of a single individual. Galileo Galilei, Italian Physicist
David Simpson, PE
MuleShoe Engineering
In questions of science, the authority of a thousand is not worth the humble reasoning of a single individual. Galileo Galilei, Italian Physicist
LittleInch
Petroleum
Emmanuel Top. I would hate to disagree with you, but my view is that the flow regime does matter. There is a great deal of difference in the instantaneous liquid velocity between level stratified / wavy flow and a slug type flow, but the analysis will tell you that on average the liquid fraction is fixed and hence on average the liquid velocity is fixed.
As you and zdas04 also note, even the average liquid velocity will change along the line as pressure changes, gradually increasing if the overall flow is steady state. I think "superficial" velocity is just the standard liquid flowrate at some fixed volume fraction. Actual is actual and will vary a lot along the line.
Remember - More details = better answers
Also: If you get a response it's polite to respond to it.
As you and zdas04 also note, even the average liquid velocity will change along the line as pressure changes, gradually increasing if the overall flow is steady state. I think "superficial" velocity is just the standard liquid flowrate at some fixed volume fraction. Actual is actual and will vary a lot along the line.
Remember - More details = better answers
Also: If you get a response it's polite to respond to it.
LittleInch,
All of the places I know of where superficial velocity is useful re-calculates it at each point in the line (I don't know of anywhere that the average over the line in multi-phase flow would be useful). I don't show gas superficial velocity in the graphic above (it isn't important to that particular segment of the class), but in the left hand example the gas superficial velocity at the bottom is around 4 ft/s and at the top it is closer to 30 ft/s. At 30 ft/s a drag model like Souder-Brown or Turner says it will drag small droplets along with the bulk flow. At 2 ft/s all the drag models say it won't.
David Simpson, PE
MuleShoe Engineering
In questions of science, the authority of a thousand is not worth the humble reasoning of a single individual. Galileo Galilei, Italian Physicist
All of the places I know of where superficial velocity is useful re-calculates it at each point in the line (I don't know of anywhere that the average over the line in multi-phase flow would be useful). I don't show gas superficial velocity in the graphic above (it isn't important to that particular segment of the class), but in the left hand example the gas superficial velocity at the bottom is around 4 ft/s and at the top it is closer to 30 ft/s. At 30 ft/s a drag model like Souder-Brown or Turner says it will drag small droplets along with the bulk flow. At 2 ft/s all the drag models say it won't.
David Simpson, PE
MuleShoe Engineering
In questions of science, the authority of a thousand is not worth the humble reasoning of a single individual. Galileo Galilei, Italian Physicist
EmmanuelTop
Chemical
LittleInch, we are basically saying the same thing. If you read carefully what I said about the actual liquid flow, you'll see that it is highly dependent on the flow regime. The point was that if one can know the actual liquid flowrate (at any point of interest), it becomes easy to calculate the actual liquid velocity, for any flow regime. The actual liquid flow can rarely be known with high degree of certainty. Hence in reality the velocity can be anywhere between zero (point of liquid accumulation) and the gas velocity (fully dispersed flow).
Dejan IVANOVIC
Process Engineer, MSChE
Dejan IVANOVIC
Process Engineer, MSChE
LittleInch
Petroleum
I did read it carefully and maybe misunderstood a little. I'm pretty sure we are all saying the same thing. Just one final point - I don't normally think of the liquid in multiphase flow to be incompressible, but more like fizzy liquid liable to change density and volume depending on pressure and containing lots of little bubbles. But I think we all get the drift.
Remember - More details = better answers
Also: If you get a response it's polite to respond to it.
Remember - More details = better answers
Also: If you get a response it's polite to respond to it.
- Status
Similar threads
- Question
