CStruc
Structural
- Oct 4, 2017
- 5
I am working on my first concrete tank design and using ACI 350-06 for the first time. I am working on the wall flexure design checks, and I thought I understood how to correctly determine/apply the Sd factor. However, I am a bit confused after looking back at this old thread from 2010:
thread507-273684
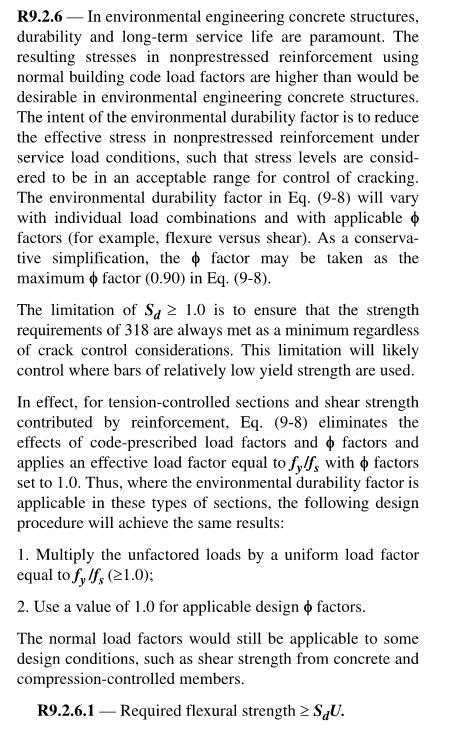
JAE indicated that a Phi of 1 (φ = 1.0) is to be used when checking against the increased moment values with Sd. Again, I am new to 350, but this is not how I understand the procedures given in chapter 9 requirements. I do see that an equivalent procedure is given in the commentary (R9.2.6, paragraph 3), which, from my understanding, can be summarized as this: Sd x U = fy/fs x (unfactored loads). But if you are going to calculate Sd using eq. 9-8, then the approach: φMn ≥ Sd*LF*(M_service) would become UNconservative if a φ=1 is used when 0.9 is needed.
Would JAE (or anyone) please give me some input or their thoughts on this.
Much appreciated!
thread507-273684
JAE indicated that a Phi of 1 (φ = 1.0) is to be used when checking against the increased moment values with Sd. Again, I am new to 350, but this is not how I understand the procedures given in chapter 9 requirements. I do see that an equivalent procedure is given in the commentary (R9.2.6, paragraph 3), which, from my understanding, can be summarized as this: Sd x U = fy/fs x (unfactored loads). But if you are going to calculate Sd using eq. 9-8, then the approach: φMn ≥ Sd*LF*(M_service) would become UNconservative if a φ=1 is used when 0.9 is needed.
Would JAE (or anyone) please give me some input or their thoughts on this.
Much appreciated!