Hi all,

I am trying to prepare an excel spreadsheet following the logic defined in 'Improved Schmidt Method for Predicting Temperature Development in Mass Concrete' by Christopher P. Bobko, Vahid Zanjani Zadeh, and Rudolf Seracino (attached).

I am obtaining unexpected results, I wondered if anyone had experience with this method or could spot my obvious error....it is driving me mad!

I am studying an element with width 1.6m. I have site thermocouple data from the curing of this element at 1 hour intervals. Therefore, to allow good comparison of the two data sets I would like the time interval in my Schmidt method to be 1 hour.

I have solved the below for a Δt = 1 hour using h = 0.00387096m^2/hour. My result is x = 0.005m (5mm). I don't really understand the significance of this result...I guess the smaller the value of x, the more computational power required..? The node spacing doesn't seem to impact the results in any other way.


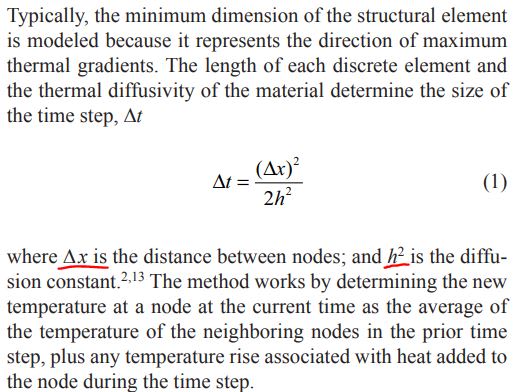
So, at each time step n, (Δt = 1 hour) and for each node, i, (spaced at 0.005m), the method requires me to average the temperature of the two adjacent nodes (call them j and k) from the previous time step (call this n-1) and then add to this the heat generated during the current time step n. The heat generated during the time step n is represented by Qh. In order to account for the ever accelerating rate of heat production in maturing concrete, it is necessary to define an 'equivalent age' for each time step and for each node. This equivalent age value t(e) is a variable within the function for Qh.

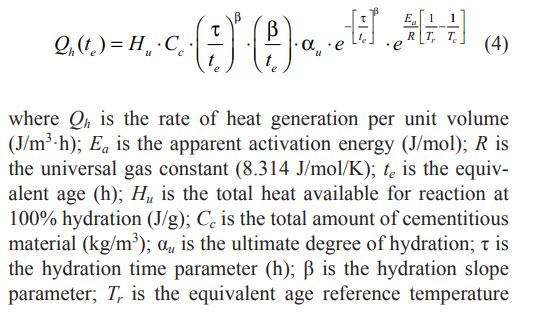
. I have determined the values of the variables alpha, beta, mu etc....I have the chemical constituents of the concrete well constrained.

By inspection of the formula for t(e), if the concrete cures above the reference temperature (taken as 293.15K) within a given time step, then t(e) increases in value. Because t(e) is larger, the value for Q(h) in that time-step is increased. This makes sense....hotter concrete will hydrate faster than cooler concrete...which in turn means that heat is generated faster. In order for this to be represented in Q(h) we have to use an equivalent rage rather than the actual age..

What isn't clear is whether the value for t(e) which I input into the function for Q(h) should be the sum of all the t(e) values for the previous time-steps? Or whether it should be the t(e) value calculated within the current time step? If I do sum the equivalent age values (t(e)), the summed value for t(e) increases very quickly and this results in unrealistic values for Qh (something like 200 Kelvin increase in temperature in one step??)..


Not such an easy topic to try and explain in words....hopefully there is someone out there who can assist. I can share my excel sheet calculations if a responder would like to take a look.

Thanks in advance,

willowman