ASCE 41-17 - Force Controlled Action
ASCE 41-17 - Force Controlled Action
(OP)
I am a little lost in understanding the intent of equation 7-35 of ASCE 41.
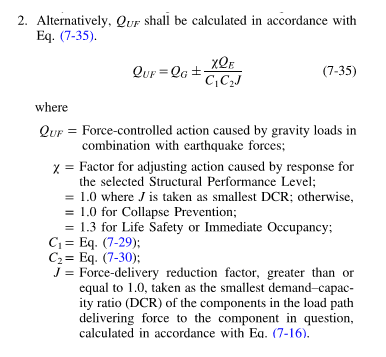
From my understanding of ASCE 41 (I have limited experience in this document), force controlled action in an element should be capable of resisting that maximum force that can be delivered to them (think seismic steel design etc) with the intent of remaining elastic.
Option (1) of 7.5.2.1.2 for determining the force to a force controlled element is worded in such a way that makes sense to me:
"the force shall be taken as the maximum action that can be developed..."
Part 2 (as snipped above above) feels odd to me. After running my calculations, I have the following factors for option 2 of the force controlled demand:
χ = 1.0
C1 = 1.79
C2 = 1.14
J = 2.0
Because C1 * C2 * J ~= 4.1 I essentially can divide my seismic force by a factor 4.1? Something feels off for an element that should be designed to remained elastic
I am checking the shear resistance of a concrete shear wall, where I have determined that I want my inelasticity to be from flexural yielding and I want my shear capacity to be able to develop the flexural yield capacity of the conrete wall.
Something feels fishy in option 2, I would at least somewhat close results between the two differing setups. Maybe I miss calc'd something somewhere.
Appreciate thoughts on the topic!
From my understanding of ASCE 41 (I have limited experience in this document), force controlled action in an element should be capable of resisting that maximum force that can be delivered to them (think seismic steel design etc) with the intent of remaining elastic.
Option (1) of 7.5.2.1.2 for determining the force to a force controlled element is worded in such a way that makes sense to me:
"the force shall be taken as the maximum action that can be developed..."
Part 2 (as snipped above above) feels odd to me. After running my calculations, I have the following factors for option 2 of the force controlled demand:
χ = 1.0
C1 = 1.79
C2 = 1.14
J = 2.0
Because C1 * C2 * J ~= 4.1 I essentially can divide my seismic force by a factor 4.1? Something feels off for an element that should be designed to remained elastic
I am checking the shear resistance of a concrete shear wall, where I have determined that I want my inelasticity to be from flexural yielding and I want my shear capacity to be able to develop the flexural yield capacity of the conrete wall.
If I run my calcs via option one I fail miserably in shear. I determine my shear demand by taking my flexural capacity and dividing by half the height of the building. This feels more in line with traditional force controlled ideology
If I run my calcs via option of of 7.5.2.1.2 I pass wonderfully
Something feels fishy in option 2, I would at least somewhat close results between the two differing setups. Maybe I miss calc'd something somewhere.
Appreciate thoughts on the topic!
S&T
Talk To Other Members
RE: ASCE 41-17 - Force Controlled Action
RE: ASCE 41-17 - Force Controlled Action
Option 1, which is the capacity design option, is there to set an upper-bound on the "real" load the force-controlled element can see. If the force-controlled element works for a capacity design, then you can stop there.
Option 2 is based on calculating the "real" force. The force in your analysis isn't your "real" force because your analysis keeps everything linear, but in real life, some elements will go nonlinear. The C1C2J factor is there to reduce your overestimated linear forces back down to "real" forces.