LR11
Structural
- Sep 13, 2001
- 166
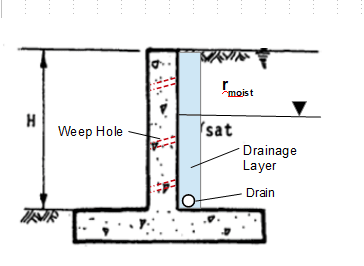
I'm looking at a retaining wall with a heel on the fill side, the heel aids significantly in sliding and rotation stability, because of the weight of the soil. I've read a number of texts to try and determine to what extent the degree of saturation reduces the effective weight of the soil, but haven't been able to find anything definite.
For a fully saturated soil, some texts mention a buoyant condition, ie, uplift. The second image below may be hypothetical, maybe not, but a saturated condition is shown on one side ... see further notes in next paragraph. At the other end of the scale, for a low degree of saturation, I would suggest that the effective weight of soil increases.
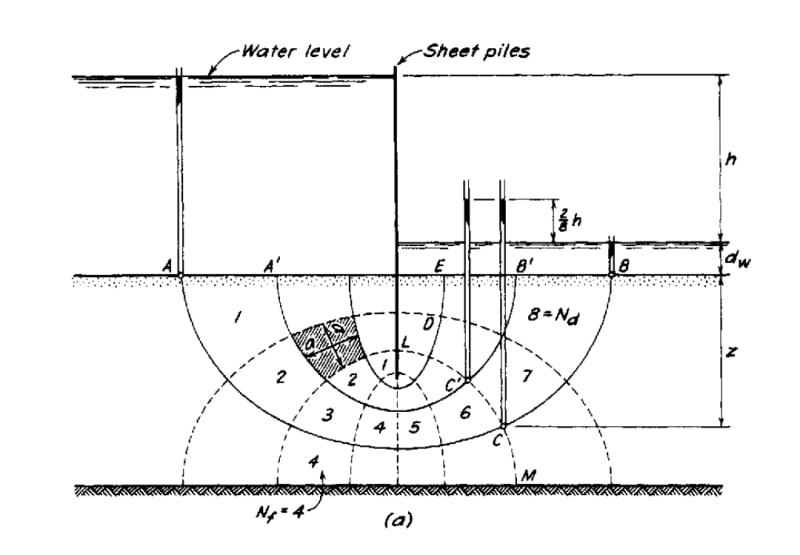
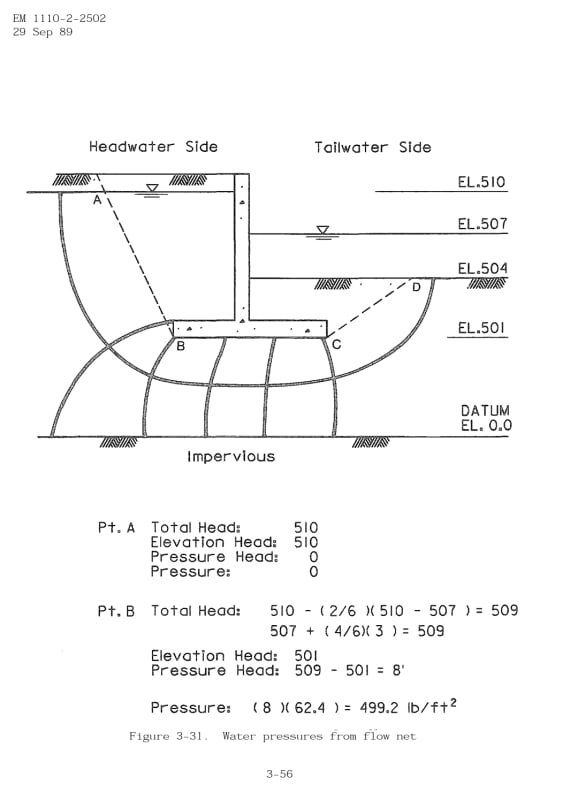
The closes I've gotten so far ... is from a text called "Foundation Design" by Wayne C. Teng, see attached extract. The text mentions that a rainfall event could cause a saturated condition, if there are no drainage measures. It mentions uplift at the surface of sliding and base of the retaining wall. It then states that you should add drainage measures for an economic design. But there is no quantification for the reduced effective soil weight. It does mention flowlines but I'm not really comfortable with extrapolating from these.
So given that I will have a drainage strip and pipe, how could I quantify the effective weight of the soil for a saturated condition.
Has anyone done this using flowlines? Or is there a rule of thumb somewhere, with the soil type as a variable.
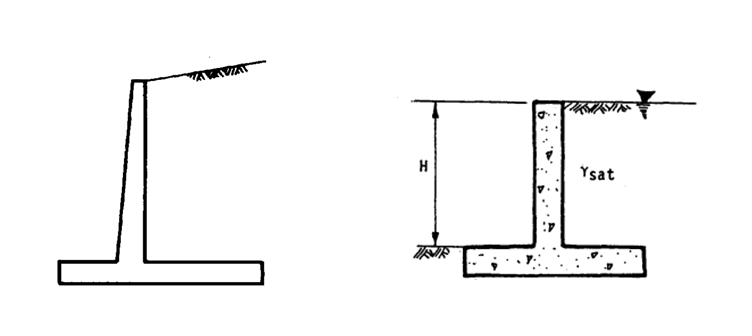
For a fully saturated soil, some texts mention a buoyant condition, ie, uplift. The second image below may be hypothetical, maybe not, but a saturated condition is shown on one side ... see further notes in next paragraph. At the other end of the scale, for a low degree of saturation, I would suggest that the effective weight of soil increases.
The closes I've gotten so far ... is from a text called "Foundation Design" by Wayne C. Teng, see attached extract. The text mentions that a rainfall event could cause a saturated condition, if there are no drainage measures. It mentions uplift at the surface of sliding and base of the retaining wall. It then states that you should add drainage measures for an economic design. But there is no quantification for the reduced effective soil weight. It does mention flowlines but I'm not really comfortable with extrapolating from these.
So given that I will have a drainage strip and pipe, how could I quantify the effective weight of the soil for a saturated condition.
Has anyone done this using flowlines? Or is there a rule of thumb somewhere, with the soil type as a variable.