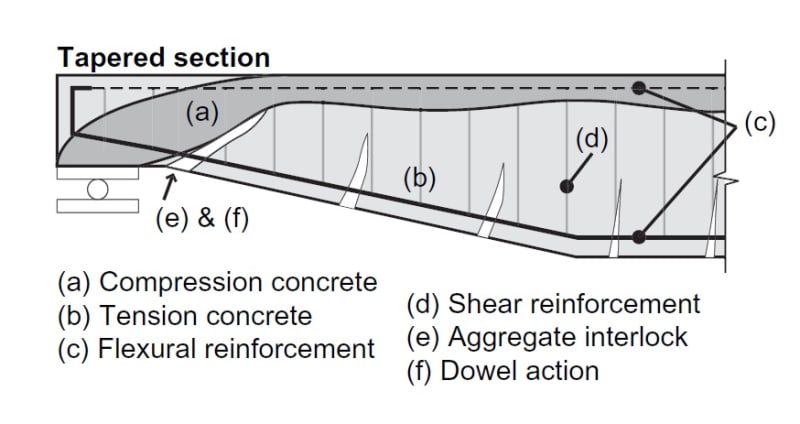
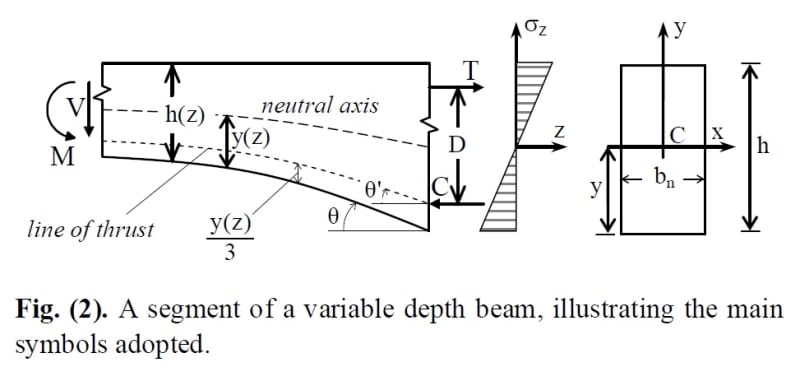
I tend to program stuff, so I'd write a program that splits the beam into 1000 little pieces and calculates the d, V, and M, and full shear design, at each little piece. It would spit the results into an Excel table. Then you can use Excel (or another program, or a subroutine of the 1000 piece program) to split it into a few sections, like 10' long each. You can even have your program design stirrup spacing and size at each of the 1000 pieces based on a set of selection rules (like use #3 down to 4" spacing, then try #4 down to 4" spacing, and so on) and the second program can grab the highest reinforcement in those sections.
The precursor to this program would be something that calculates V and M based on loading and end conditions, and splits it into an arbitrary number of pieces. I made something for that as well, which took like 2 days, but it's very useful to build off of.
My personal way is to not use formulas and derivations anymore. If you're proficient in scripting/coding, it's less work to just automate it. On the other hand, if the coding skills aren't there, your way with making a new equation would be better. I can see it getting really tricky to slam a varying triangular or parabolic d into the stirrup formula, especially if you use the formula that also includes moment (in ACI code).